HABITATS CAN TRANSPORT perhaps to Mars

Habitats could comfortably transport large human populations
via orbits throughout the Solar System.
Simulate earth gravity via continuous spin around longitudinal axis.
Large external mirrors could reflect sunlight into habitat
to provide power for production and life support requirements.
Island-3 habitats (r = 3,600 m, est. l= 7.2 km) has about 40,000 acres
of surface area for living quarters, agriculture and other needs.

Habitats can transfer payloads to Mars via transfer orbits. 

Once there, habitats could house Martian humans in orbits about Mars.

Martian habitats could even share same Martian orbits as Martian moons.

HUMANS CAN ORBIT MARS IN HABITATS
Deimos and Phobos Two moons of Mars could serve as operational bases and likely provide considerable construction materials. Many agricultural items produced in habitat can transfer to Martian surface; such items can help terraform Mars over many years. Eventually, Mars (both surface and subsurface) will prove to be an expansive source of agriculture and raw materials.  However, Mars is unlikely to ever reproduce Earth like gravity; longitudinal spin of the cylindrical habitats can produce 1-g via centrifugal force.	Martian humans will always prefer to live in the large orbiting habitats; conditions will be more "Earth like" and access to interplanetary travel will be much easier than for the Martian humans who choose to reside on Mars itself. In contrast with living submerged under Martian surface, orbiting habitats provide a ready made, comfortable and controlled environment. Agriculture flora would produce oxygen and food fauna for petting and food Simulated Earth Gravity from longitudinal spin of cylindrical habitat. Energy Source sunlight from large exterior mirrors which never tarnish. Before habitat orbits Mars, habitat has to get there.
USE HOHMANN TRANSFER (HT) FROM EARTH TO MARS.
PROBLEM: During lengthy space voyages, humans require plentiful supplies: food, water, oxygen as well as Earth like gravity.  None of those are now available in current space vehicles. Infrequent, interplanetary flights are now accomplished by Artificial Intelligence (AI) devices inside small capsules. Sending humans in such vessels seems pointless; not only would travelers have to endure months/years of 0-g conditions which would likely permanently disable them; but providing required consumables would be challenging at best. SOLUTION: On the other hand, interplanetary flight conditions could greatly improve if humans traveled on habitats during these lengthy missions. Habitats can be very comfortable "homes" during lengthy flights. Earth Pos. ∠ⴲ Hab. Pos. ∠Hab Cumm. Time T Mars Pos. ∠♂ 0° 0° 0 dy 57.3° 49.9° 53° 50 dy 83.6° 99.9° 99° 100 dy 109.9° 149.9° 126° 150 dy 136.2° 199.8° 151° 200 dy 162.5° 258.6° 180° 259 dy 180.0° T×.999° day Est. Σt 57.3°+T×.526° day Power supply via sunlight from large external mirrors.   Onboard water stores and agriculture provide oxygen and food. Centrifugal force from longitudinal spin provides 1-g gravity. Large human population provides essential social interaction. Constant communication with "home planet" reinforces purpose. As a matter of fact, habitats might be necessary for the several years required for trips to other planetary systems: Jupiter and moons, Saturn and rings and moons, Uranus, Neptune, perhaps even the planetoids such as: Pluto, Ceres, etc.	GETTING THERE Transfer Orbit (TO) is a highly eccentric orbit between two planetary orbits. A non-powered object must use a transfer orbit to travel between planetary orbits. The most efficient transfer orbit is a Hohmann Transfer (HT), which uses least possible fuel  for "burns" to enter/exit TOs between orbits. For HT from Earth to Mars, the perihelion (nearest point to Sol) is the exit point from Earth's orbit and its aphelion (farthest point from Sol) is where it intercepts Mars.
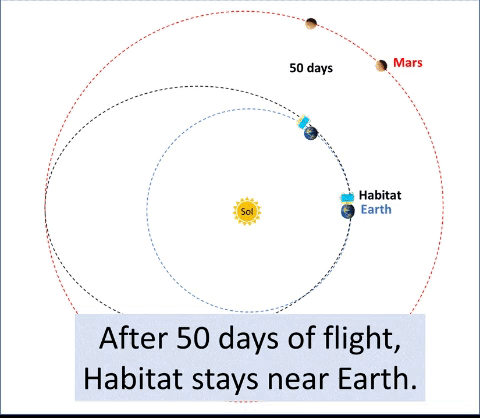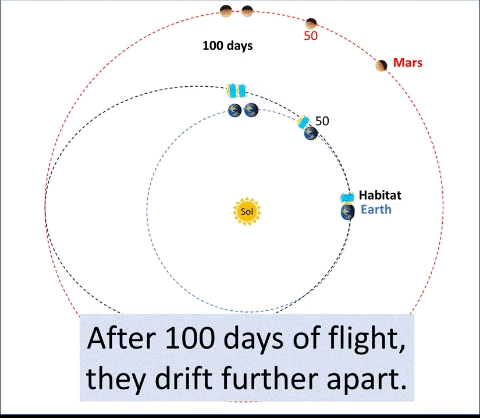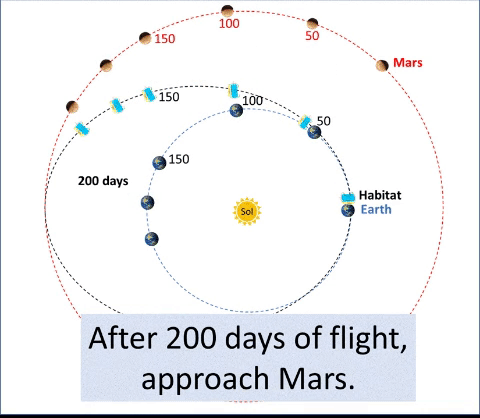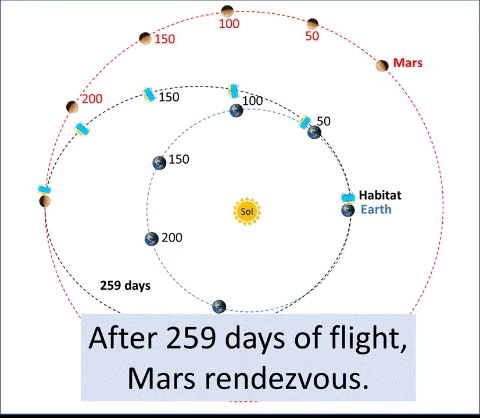HT APHELION (QHT) AND HT PERIHELION (qHT)
HT Aphelion (QHT) is the semi-major axis of Mars, a♂. QHT = a♂	HT Perihelion (qHT) is semi-major axis of Earth, aⴲ. qHT = aⴲ = 1.0 AU
After 259 days of unpowered flight, habitat reaches destination aphelion. Vehicle emits a controlled thrust to orbit destination planet.	At departure perihelion, vehicle emits controlled thrust to exit Earth's orbit and enter transfer orbit. Transfer must begin when properly planned.
SEMI-ORBIT GIVES TRANSFER TIME
From QHT and qHT, compute semi-major axis of transfer orbit, aHT Semi-major axis: aHT = QHT + qHT 2 = a♂ + 1  2 = 1.26 AU From Kepler's Third Law, compute PHT , period of transfer orbit, Period of Orbit PHT = √[aHT]3 = 1.4143 year Axiomatic: Transfer time is the duration, THT, of the semi-orbit from qHT to QHT. Transfer Time: THT  =  PHT  2 × 365.25 days  year  = 258.3 days	From QHT and qHT , compute: ℓ and e: Semi-latus rectum: ℓ = 2×Q×q Q + q = 1.20635 AU Eccentricity: e = Q - q Q + q = 0.20635 For reference (θ = 0°), consider a ray from Sol to perihelion, qHT. As Habitat orbits from qHT  to QHT, angle, θ, goes from 0° to 180°. Compute radius, distance from Sol to Habitat, for each angle, θ. Rθ = ℓ 1 + e×Cos(θ) In the plane of the Transfer Orbit, define a 2 dimensional (X,Y) coordinate for each θ.Use Sol as origin (0,0). θ R X Y Deg AU AU AU 0° 1.000000 1.000000 0.000000 1° 1.000026 0.999874 0.017453 Given ℓ 1+e×Cos(θ) R×Cos(θ) R×Sin(θ)
APPROXIMATE INCREMENTALS
Use Pythagoras to approximate  incremental distances between each coordinate [distance between (Xθ-1,Yθ-1) and (Xθ,Yθ) ] Δxθ = xθ - xθ-1 Δyθ =  yθ - yθ-1 dθ = √[(Δxθ)2 + (Δyθ)2] Convert distance from AU to kilometers. θ X Y dθ Deg AU AU AU km 0° 1.000000 0.000000 n/a n/a 1° 0.999874 0.017453 0.017453 2,610,979 2° 0.999495 0.034903 0.017454 2,611,138 Given R×Cos(θ) R×Sin(θ) AU = 149,597,870.7 km	Use point velocity to approximate incremental travel times. Velocity for any angle, θ. μSol ≈ 887.123 AU-km2 Vθ = √ [ μSol × ( 2 Rθ - 1 a )] Time between each coordinate [between (Xθ-1,Yθ-1) and (Xθ,Yθ) ] θ X Y dθ Vθ tθ Deg AU AU km km/sec day 0° 1.000000 0.000000 n/a n/a n/a 1° 0.999874 0.017453 2,610,979 34.239 0.8826 2° 0.999495 0.034903 2,611,138 34.237 0.8827 3° 0.998864 0.052348 2,611,457 34.233 0.8829 Given R×Cos(θ) R×Sin(θ) dθ 86,400×V

PROGRESS BY DEGREES
θ	Rθ	Vθ	Xθ	Yθ	dθ	dkm	tθ	Tθ	Table shows total travel time for selected angle, theta,(θ), of Hohmann Transfer from Earth to Mars BACKGROUND: Hohmann Transfers (HTs) are the most energy efficient transfer orbits. Hohmann transfer time is easily calculated. THT = (1 + a♂)3/2 5.656 yr A.E. Roy Orbital Motion pg. 354 For convenience,  TE chooses to use HT time as a typical transfer orbit duration.
Deg	AU	km/sec	AU	AU	incr. AU	incr. km	incr. days	cumm. days
0°	1.000	34.239 kps	1.0000	0.0000	n/a	n/a	n/a	n/a
1°	0.9998	34.238 kps	0.9998	0.0174	0.0174	2,610,979	0.92	0.92
2°	0.9994	34.237 kps	0.9994	0.0349	0.0174	2,611,138	0.92	1.85
3°	0.9988	34.233 kps	0.9988	0.0523	0.0174	2,611,457	0.92	2.77
. . .	. . .	. . .	. . .	. . .	. . .	. . .	. . .	. . .	Cumulative Times Approximate total travel time for any angular position during transfer. From perihelion(θ=0°) to 45°, 90° and 135°; with respective durations of 43, 95 and 167 days .
45°	1.0527	32.908  kps	0.744	0.744	0.0185	2,767,364	1.02	43.03
90°	1.2063	29.448 kps	0.000	1.206	0.0215	3,210,053	1.34	95.46
135°	1.4120	25.523 kps	-0.999	0.999	0.0249	3,736,381	1.84	166.97
. . .	. . .	. . .	. . .	. . .	. . .	. . .	. . .	. . .
177°	1.5195	23.710 kps	-1.517	0.079	0.02652	3,967,169	2.13	251.96	Previous tables compute incremental distance/time for each degree of habitat's transfer. This table has examples for a few angles of cumulative travel times as shown. Source table has cumulative values for θ = 0° to 180°. Kepler's Equation uses an elegant method for more accurate travel times.
178°	1.598	23.712 kps	-1.518	0.053	0.02652	3,967,866	2.13	254.09
179°	1.599	23.715  kps	-1.519	0.026	0.02652	3,968,331	2.13	256.22
180°	1.5200	23.718  kps	-1.520	0.000	0.02653	3,968,564	2.13	258.35
Given	ℓ 1+e×cos(θ)	√[ μSol ( 2 Rθ - 1 a )]	Rθ × cos(θ)	Rθ × sin(θ)	√[(Δx)2+(Δy2)]	dθ × (km/AU) 1 AU = 149,597,871km	dkm÷Vθ	Σtθ

Ideal transfer orbit goes directly from Earth to Mars. However, it requires a precisely timed "launch window" of a few hours once every 758 days. To do this, habitat must enter Hohmann Transfer (HT) exactly as Mars leads Earth by 52.7°.   This precise position happens one time during each Earth-Mars Synodic orbit (about 758 days). This is a great opportunity for that one day out of 758;  however, this requirement seems to prevent travel to Mars during the other 757 days. TE considers this to be too restrictive.

To slightly expand launch window, consider a "Parking Orbit (PO)".

EXAMPLE: If habitat departs Earth two days late, it intercepts Mars orbit about one degree behind the planet Mars (about 4 million kilometers).  If habitat remains on Mars orbit, it forever lags Mars by 4 million km.  To catch up with Mars, habitat could instead intercept a slightly smaller, concentric orbit.  In this slightly quicker PO, habitat slowly decreases angular distance to Mars. PO is a good "last resort" if fuel is limited (which is why we bother to wait for the HT "launch window".)

1) Semimajor axis (a)

Orbit aAU akm Earth 1.00 AU 149,597,871 km PO 1.50 AU 224,396,806 km Mars 1.52 AU 227,388,764 km Given CAU×149,597,871km/AU a♂ - aPO  = 2,991,958 km For circular orbit, a is constant distant from Sol. For Mars, a♂ is 1.52 Astronomical Units (AU). Let parking orbit (PO), aPO , be 1.50 AU. Assume orbits (Mars and PO) to be concentric and about 3 million kilometers apart. Object in inner orbit (PO) will travel slightly faster than Mars.
2) Circumference (C)
Orbit Semi- Major Axis Circumference 1° arc Dist. a CAU Ckm Δkm Earth 1.00 AU 6.283 AU 939,923,423 km 2,610,898 km PO 1.50 AU 9.424 AU 1,409,926,718 km 3,916,347 km Mars 1.52 AU 9.550 AU 1,428,725,740 km 3,968,682 km Given 2 π a CAU×149,597,871km/AU Ckm/360° For circular orbits, approximate C: C= 2 π  a   For PO, one degree arc distance: Δkm = CPO ÷ 360 Δkm =  3,916,463 km
3) Period (P)
Orbit Semi-Major Axis Period 1° arc Time a PYr Pdy Δdy Earth 1.00 AU 1.000 Yr 365.25 days 1.015 dy PO 1.50 AU 1.837 Yr 671.0 days 1.864 dy Mars 1.52 AU 1.874 Yr 684.5 days 1.901 dy Given √a3 PYe × 365.25 dy/Yr Pdy/360° For circular orbits, approximate P: P= √a3  For PO, one degree arc time: Δdy = PPO ÷ 360 Δdy =  1.864 days

4) Orbital Velocity: Linear (V)

Orbit Semi-Major Axis Circumference Period Linear Velocity a CAU Ckm PYr Pdy Vkm/day Vkm/sec Earth 1.00 AU 6.283 AU    939,951,145 km 1.000 Yr 365.25 days 2,573,456 kpd   29.79 kps PO 1.50 AU 9.424 AU 1,409,926,718 km 1.837 Yr 671.0 days 2,101,232 kpd   24.31 kps Mars 1.52 AU 9.550 AU 1,428,725,740 km 1.874 Yr 684.5 days 2,087,223 kpd 24.16 kps Given 2 π a CAU×149,597,871km/AU √a3 PYr×365.25 dy/Yr Ckm÷Pdy 29.785/√a V=  √[ μSol ( 2 Rθ - 1 a )] Adjust for circular orbit, Rθ = a; and assume: μSol ≈  887 AU-km2/sec2 V = √[ 887.13 AU-km2/sec2 a ] = 29.785 km/sec √a

Even when aligned with Mars, Habitat will still be in smaller orbit and displaced from Mars about 3 million kilometers.
Perhaps, flight planners could use following maneuver:

1) During the 91 day catch up period, Space Tug could gradually nudge Habitat  closer to Martian orbit, perhaps daily nudges are best.

2) For each daily nudge, the habit assumes a slightly larger orbit and thus closer to Mars orbit, unfortunate when Habitat's orbit gets slightly larger, it also gets slightly slower with an even smaller daily differential.  Thus, total transit time (from PO to MO) might take 100 days.

---

CONCLUSION: HT and subsequent PO might be a good "last resort" for limited fuel scenarios.
However, if fuel is available, compute direct transfer orbit instead of HT.

---

---

SLIDESHOW

---

---

---

---

VOLUME O: ELEVATIONAL
VOLUME I: ASTEROIDAL
VOLUME II: INTERPLANETARY
VOLUME III: INTERSTELLAR

---

---

---

---

5) Orbital Velocity: Angular (ω)

Compute angular velocity directly from a, semimajor axis. Divide total circumference angle (360°) by orbital period (in days). CDeg ÷ PPO = 360° ÷ 671.0 days = 0.537°/day Reduce above tables to: Orbit Semimajor Axis Angular Velocity aAU ω Earth 1.00 AU 0.999°/day PO 1.50 AU 0.537°/day Mars 1.52 AU 0.526°/day Given 360°  √a3 ×365.25 dys/yr Differential Closure (δ) With Habitat in the smaller, quicker orbit, it will daily decrement difference between Habitat in Parking Orbit and Mars in its orbit. δ = ωPO - ω♂ δ = 0.537°/day - 0.526°/day = 0.011°/day If Habitat is 1° behind Mars, Habitat will completely align with Mars in about 91 days. TAlign = 1°/δ TAlign = 1° ÷ .011°/dy= 91 days