ACCELERATE FOR A DAY

CONTENT FINAL VELOCITY AVERAGE VELOCITYDISTANCE TRAVELEDDAILY RATE: KPS/DAYDAILY RATE: AU/DAYDAILY RATE: %c/DAYSSIDEBAR: GALILEO

Albert Einstein loved thought experiments.

---

EQUIVALENCE: Perhaps the best known thought experiment uses an elevator which accelerates at same rate as a free falling object near Earth's surface. The occupants can't ascertain whether the elevator is accelerating in space or static on Earth's surface; thus, THE EQUIVALENCE PRINCIPLE. 

"force at a distance". In the late 1600's, Newton realized that objects remain at constant velocity until a force acts on them to change their velocity. He further discovered that gravity (attraction between masses) acts as a force at a distance and causes objects to accelerate. Much later, Albert Einstein gave considerable thought to the accelerating elevator concept and realized that occupants inside the elevator would not discern whether that elevator was static on Earth or accelerating at g in space. Either way, they'd be pressed against the floor by same force which gives us our weight on Earth's surface. For example, a dropped ball would fall at same rate with same trajectory. Thus, Thought Experiments quickly led Einstein to conclude that gravity and acceleration are closely related. (Note: Acceleration is one of the basic units of motion.) Perhaps we can slightly modify Einstein's experiment and replace the elevator with a notional spaceship which can freely accelerate at rate g throughout the Solar System. Because the spaceship occupants will experience simulated gravity throughout this flight, we'll take the liberty of calling this "g-force" acceleration.

FINAL VELOCITY VFin = t × 10 m sec² Elapsed Time Final Velocity t VFin 1 sec 10 m/sec 2 sec 20 m/sec 3 sec 30 m/sec .. ...... 10 sec 100 m/sec 100 sec 1 km/sec 1,000 sec 10 km/sec Given g × t Define acceleration as velocity per time: a = v/t Determine velocity with well known equation: v = a × t thus, velocity equals acceleration times duration. Let a = g (= 10m/sec2); then, readily compute values (see Table). Constant g-force increases velocity at a linear rate; achieve final velocity (VFin) after elapsed time of t seconds.

AVERAGE VELOCITY To determine the average speed of a constantly accelerating object, we can use half of the final velocity if and only if our acceleration complies with the following conditions: 1. Start at rest, or initial velocity equals 0 vIni = 0 m/s 2. Acceleration is constant, or velocity increases at steady rate a = 10 m/sec2 = g VAve =VFin / 2 Elapsed Time Final Velocity Ave. Vel. t VFin VAve 1 sec 10 m/sec 5 m/sec 10 sec 100 m/sec 50 m/sec 100 sec 1.0 km/sec 0.5 km/sec 1,000 sec 10 km/sec 5.0 km/sec 3,600 sec (= 1 hr) 36 km/sec 18 km/sec 86,400 sec (=24hr= 1 day) 864 km/sec 432 km/sec Given g × t VFin/2 Thus, we achieve average velocity exactly halfway through the acceleration interval; so, compute average velocity by dividing final speed by two. VAve = VFin 2 Given constant acceleration and initial velocity of zero, average velocity for any duration is half of final velocity.

Galileo's most famous experiment at the Leaning Tower of Pisa showed that heavier objects fall at the same rate as lighter objects. In fact, he did numerous experiments in a more practical fashion, rolling balls down sloping troughs at different angles. He discovered that an object's free-fall velocity varies with time, not with mass. In fact, numerous observations have determined that an object's speed increases (accelerates) as the free-fall time increases. Precise observations have determined this acceleration rate to be 9.80665 meters per second per second (m/sec2); for convenience, our thought experiment will round this value to 10 m/sec2. Thus, we now know that near Earth's surface, an object increases its downward speed an additional 10 m/s for every second of free-fall. After 2 seconds of free-fall, object's speed is 20 m/s; after 3 seconds, 30 m/s; and so on.  If the shape of an object interferes with the fall (like a feather or a parachute), then air resistance acts as a counterforce to impede the gravitational force for much slower speed. SUMMARY: Friction free objects with different masses (such as a small ball bearing and a much larger bowling ball) increase velocity a the rate of g, 10 meters per sec for every second of free fall, or spacecraft increases velocity for every second of g-force powered spaceflight.

Galileo Gallilei was born in Pisa, Italy on February 15, 1564. His family were of nobility but not rich. In 1581, Galileo began studying at the University of Pisa, where he learned Aristotelian physics which held that heavier objects fall faster through a medium than lighter ones. Galileo eventually disproved this idea and asserted that all objects, regardless of density, fall at the same rate in a vacuum. To determine this rate, Galileo performed various experiments with dropped objects from a certain height. In an early experiment, he rolled balls down a gently inclined plane; then, he determined their positions after equal time intervals. He wrote down his discoveries about motion in his book, De Motu, "On Motion." DISTANCE TRAVELED d = t × VAve Elapsed Time Final Speed Ave. Speed Total Dist. t VFin VAve d 1 sec 10 m/s 5 m/s 5 m 2 sec 20 m/s 10 m/s 20 m 3 sec 30 m/s 15 m/s 45 m . . . ... ... ... 10 sec 100 m/s 50 m/s 500 m Given g × t Vfin/2 t × Vave g×t g×t/2 g×t2/2 Gravity accelerates freely falling objects 10 meters per second for every second of flight. After 3 sec, object's speed is 30 m/sec. However, the distance fallen will reflect object's average speed. For example, it falls 5 meters in first second of free fall due to its average speed of 5 meters per second. After three seconds, the object achieves an average speed (VAve) of 15 meters per second; multiplying this value by 3 seconds duration gives us 45 meters distance. ALTERNATE DISTANCE METHOD Since 10m=.01km, let g = .01 km/sec2 t t2 d Elapsed Time Total Dist. 10 sec 100  sec2 .5 km 1 min (=60 sec) 3,600 sec2 18 km 1 hour (=3,600 sec) 1.296×106 sec2 64,800 km 1 day (=86,400 sec) 7.464×109 sec2 37,324,800 km t t2 g × t2 /2 Determine distance traveled with another simple equation. Recall: VAve = VFin/2 Thus, d= VAve × t = t(VFin/2). Recall: VFin = at d = t(VFin/2) = t (a × t/2) d = (a × t2)/2. Use a = g = .01 km/sec2; then, g-force distances at can be determined:  d = g × t2/2 Note that final data row uses elapsed time for an entire day (86,400 sec); thus, g-force distance for an entire day.  Galileo’s most famous invention was the telescope. In 1609, Galileo’s first telescope was modeled after previous telescopes that could magnify images three times. He soon created another telescope which could magnify twenty times. With this improved telescope, he could observe features on the moon,  observe a supernova, verify the phases of Venus, discover sunspots and discover the four largest satellites of Jupiter. His discoveries confirmed the Copernican system; Earth and other planets revolve around the Sun. This starkly contrasted with the view of the Church which then held the Universe to be geocentric. Galileo's belief in the Copernican System eventually got him into trouble with the Catholic Church’s Inquisition which was charged with the eradication of heresies. A Church committee of consultants declared this Copernican proposition to be heretical. Because Galileo supported the Copernican system, he was warned to not discuss or defend Copernican theories.

In 1624, Galileo understood from Pope Urban VIII that he could write about Copernican theory as long as he treated it as a mathematical proposition; thus, he felt safe to publish Dialogue Concerning the Two Chief World Systems. However, certain mean spirited enemies of Galileo persuaded the Pope to interpret certain passages as personal insults. Thus, Galileo was called to Rome in 1633 to face the Inquisition. Galileo was found guilty of heresy for his Dialogue …, and was sent to his home near Florence to be under house arrest for the rest of his life. Though disappointed by the verdict, this distraction free environment enabled Galileo to produce yet more scientific work. In 1642, Galileo died at home. Isaac Newton was born the same year.	Express g differently g = 10 m sec2 × 1 km 1000 m g = .01 kps sec × 86,400 sec day g = 864 kps day Kilometers per second per day After 86,400 seconds (one day) of g-force acceleration: Vfin = g * t = 864 km/sec For every day of constant acceleration, g; spacecraft increases its velocity another 864 km/sec. Thus, we can restate g as a daily rate. g = 864 km per sec / day Thus, at end of 2nd day of spaceflight, VFin = t×864km/sec/day Elapsed Time Final Velocity t VFin 1 day 864 kps 2 day 1,728 kps 3 day 2,592 kps .. ...... 10 day 8,640 kps Given g × t Vfin = g * t  Vfin = 864 km/sec /day * 2 days Vfin = 1,728 km/sec Table shows other values. Why express g as "km/sec /day"? Quickly determine inflight velocities as kilometers per second (kps), typically used for orbital velocities of Solar System objects (planets, asteroids, comets). For example, Earth's orbital velocity around Sol is about 30 kps.  NOTE: After just one day of g-force acceleration, spacecraft attains tremendous speed many times this.
Astronomical Units/day per day For an even more convenient unit of g, consider the Astronomical Unit (AU), average distance from Earth to Sol. Distance to nearby planets is conveniently measured in AUs  Exact AU = 149,597,870 km; for convenience, thought experiment rounds AU to 150,000,000 km. Express g differently g = 864 kps day × 86,400 sec day g = 74,649,600 km day2 × 1 AU 149,597,870 km g ≈ .5 AU day2  g = .5 AU/day2 Different g expression enables different velocity expressions. After one day of g-force acceleration: VFin = t × g VFin = 1 day × .5 AU/day2 VFin = .5 AU/day VAve = VFin /2 VAve = .5 AU/day ÷ 2 VAve = .25 AU/day Elapsed Time Final Speed Average Speed Total Distance t VFin VAve d 1 day .5 AU/dy .25 AU/dy .25 AU 2 day 1.0 AU/dy .5 AU/dy 1.0 AU 3 day 1.5 AU/dy .75 AU/dy 2.25 AU . . . ... ... ... 10 day 5.0 AU/dy 2.5 AU/dy 25 AU Given g × t VFin/2 t × VAve g×t g×t/2 g×t2/2   Interplanetary g-force flights will be quick, a matter of days. Therefore,  g = 0.5 AU/day2 might be a convenient expression.	Galileo's Falling Motion Experiment Galileo showed that the free-fall motion of an object has a constant acceleration. Starting from rest, distances increase in proportion to the square of the elapsed time. Since a vertical fall was too fast for Galileo to measure accurately, he slowed it down by making the ball roll down an inclined board. Across the board, along to its surface, he strung a number of taut horizontal wires, making the ball sound a click whenever it jumped over one of them. Galileo then moved the wires up and down the board, until the clicks sounded evenly spaced. If the acceleration is now a (less than near Earth gravitational acceleration, g=10m/sec/sec) and the time, t, was measured in clicks. Starting with the ball at rest (D0 = 0), one measures following: After one click, D1 = 1 unit After two clicks, D2= 4 units After three clicks, D3= 9 units After x clicks, Dx= x2 units Galileo confirmed the ratio between the distances to be that of the squares: 1, 4, 9, 16, 25… If one converts clicks into second and accounts for the angle of the board as well as for the rolling friction of the ball (not available in Galileo's day), one could even calculate g from a. For more on this experiment, see the book "The God Particle" by Nobel prize winner, Leon Lederman, with Dick Teresi.
Percent Light Speed per day Finally, consider g in terms of %c, percent light speed. Express light speed, c, differently c = 299,792.458 km sec × 86,400 sec day c = 299,792.5×86,400km day × 1 AU 149,597,870 km c ≈ 173.145 AU day First, convert c from the well known kilometers per second to AUs per day. Express g in terms of c g ≈ 10 m sec × sec = .5 AU day × day g ≈ .5 AU/day day × c 173.145 AU/day g ≈ .00289 c day = .289% c day Recall that g-force acceleration equals 0.5 AU/day; thus, we can readily transform this value into a percentage of light speed. Recall that %c is a velocity, and g is an acceleration (velocity per unit time); thus, another value for g is 0.289%c/day. Do we care about our spacecraft's light speed?? Relativistic effects might be a concern for very fast inflight speeds.	VFin = t × .289%c/day Elapsed Time Final Velocity t VFin 1 day 0.289%c 2 day 0.578%c 3 day 0.867%c .. ...... 10 day 2.890%c Given g × t 1. For interplanetary flights, we don't care about relativistic effects. Even at g-force acceleration, ship's speed stays less then 10% c throughout the Solar System. Such flights will take a few days, and table shows that we don't even reach 1% c until after three days of g-force flight. NOTE: Due to relativistic effects, our g-force spaceship will never ever reach light speed, c; even though, it can get ever closer and closer. Thus, short time measures of g-force accelerated velocities can be approximated by adding (as shown in table).  However, accuracy requires different means of calculation for longer duration flights. 2. On the other hand, relativity will definitely affect interstellar flights. Even with g-force acceleration, those flights will take years. After 300 days of g-force acceleration, we will have not even approached the Oort Cloud, but we speculate that spaceship's velocity will be .866c. At this relativistic speed, spaceship's mass doubles, and inflight time shrinks by 50%. By the time, we'll doing interstellar missions, we'll likely have some solutions for this phenomena.

Equivalent Expressions of g

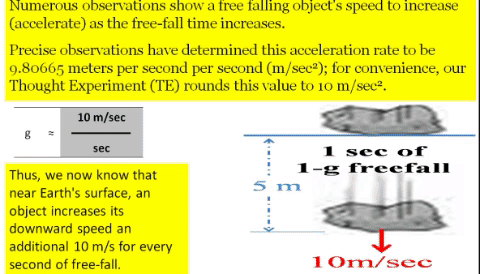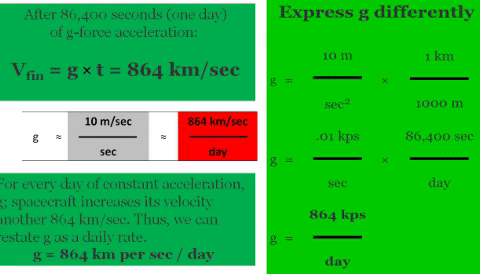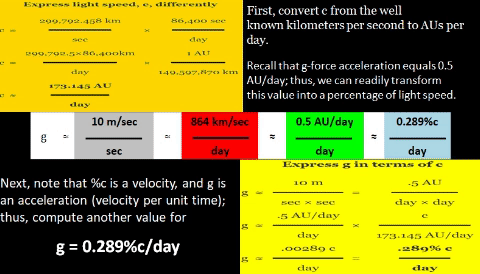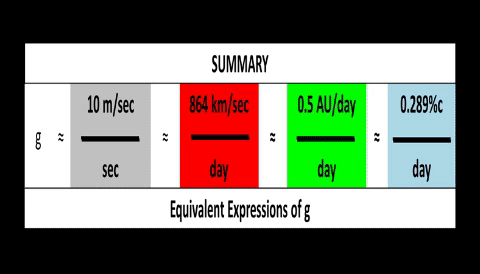
Above expressions have nearly equal values; all approximate g, acceleration due to Earth surface gravity.  Recall that g is an acceleration which is velocity per time. Above expressions are all stated as velocity per unit time.

SLIDESHOW.

---

---

---

---

VOLUME 0: ELEVATIONAL
VOLUME I: ASTEROIDAL
VOLUME II: INTERPLANETARY
VOLUME III: INTERSTELLAR

---

---

---

---