BEST OF BREED ION DRIVE: VASIMR

See following topics: Compare Plasma with ChemicalMomentum Exchange EquationThree Ways of TravelingPROPULSION EFFECT TABLE1. Exhaust Particle Velocity2. Ship Acceleration3. Ship Mass4. Relativity Factor5. Efficiency Factor6. Daily Difference7. Trip Time8. Total DifferenceAjustable Fuel Flow

Current Leading Technology: Ion Thrusters

A typical ion drive converts gas (i.e., argon, xenon, or hydrogen) into super heated plasma. To expel high speed ions out of exhaust, a magnetic nozzle directs the ion motion into useful linear momentum. Ions accelerate to perhaps 50 kilometers per second (about .000167 c). While this exhaust velocity far exceeds speeds achieved by exhaust particles from traditional chemical fueled vehicles, it is not nearly enough to produce g-force throughout a trip to neighbor planets.

---

However, an ion thruster might efficiently inject ions into Thought Experiment's (TE's) on board  particle accelerator propulsion system. The best ion thruster might be the VASIMR plasma drive, now under development by the Ad Astra Rocket Com.

---



Franklin Chang-Diaz

U.S. astronaut-scientist-entrepreneur,  Franklin Chang-Diaz leads a team which developed a revolutionary spacecraft propulsion. His company, Ad Astra, now builds the Variable Specific Impulse Magneto-plasma Rocket (VASIMR).  A very different type of propulsion system, VASIMR uses plasma to propel spacecraft.

---

Dr. Chang-Diaz explains the plasma drive: “...rocket engine of the future. As plasma is released through an exhaust nozzle, it creates the rocket effect and pushes the engine (in opposite direction). ... a plasma engine is more efficient and faster. In fact, a plasma-driven rocket could push a cargo vehicle from Earth to Mars in ninety days, about twice as fast as solid or liquid (i.e., chemicals) fueled rockets.”

---

---

---

Assume VASIMR Goes to Mars BACKGROUND: Recall Chemical Operations. Current design limitations constrain a chemical fueled rocket to short period "burns". Therefore, one short chemical burst at beginning of flight will speed the vessel to an orbital path to the destination; another short burst at end of voyage slows the vessel to adjust speed with destination orbit. With no additional thrust during the flight, the vessel must stay in a carefully planned Solar orbit for most of the flight. Plasma Performance is Better. Reason for plasma's improved performance, plasma engine constantly propels spacecraft (via plasma ion exhaust) throughout the voyage; this achieves greater speed and does not constrain the vessel to an orbital path.	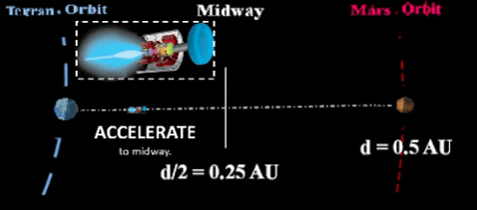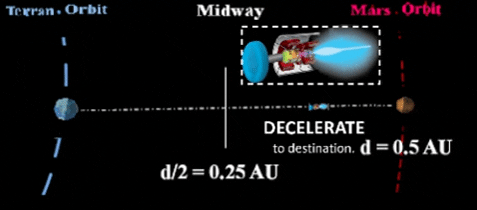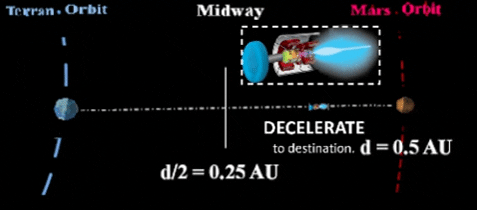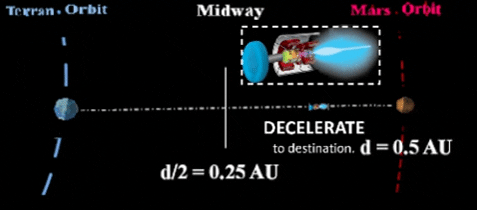Constant VASIMR Acceleration Assume midway slowdown. Minimum Distance from Earth to Mars at opposition. d ≈ .5 AU ≈ 75,000,000 km Acceleration Distance. Midway. dAcc =  d/2 dAcc ≈ 37,500,000 km ≈ .25 AU Deceleration Distance. Assume equals acceleration distance. d = 2 × dAcc = dDec + dAcc dDec ≈ .25 AU ≈ dAcc Typical Time. Assume typical trip time is 3 months. t = 90 day t = 7.776×106sec Acceleration Time Axiomatic: half of total time. tAcc = t/2 = tDec tAcc = 3.888×106sec Average Velocity (NOTE: Assume initial velocity = 0.) Divide total distance (d) by total time (t). VAve = dAcc tAcc = 37.5×106km 3.888×106sec VAve = 9.65 km/sec
Typical VASIMR Acceleration Acceleration (Newtonian: d= a × t2/2.)  VASIMR acceleration (.005 m/sec2) is much less than g-force acceleration, 9.8065 m/sec2. (i.e. "microgravity") a = 2×dAcc (tAcc)2 = 2×(37.5×106km) (3.89×106sec)2 a = 4.96×10-6 km sec² Max Velocity mid-time: (tAcc=45 day) mid-dist. (dAcc=.25 AU). VMax = a × tAcc VMax = 4.96×10-6 km sec² × 3.888×106 sec VMax = 19.2845 km/sec CONCLUSION: After 45 days of VASIMR acceleration, ship's max velocity is about same as a typical asteroid's orbital velocity.	Helicon Heats Gases Helicon's radio waves heat gases to plasma state (millions of degrees). Thus, rocket performance improves with hotter exhaust; thrust greatly exceeds that of chemical reactants which only reach thousands of degrees in a conventional rocket engine. Thrust from the plasma engine could boost a spacecraft for a longer time and with better efficiency than conventional engines. Plasma engines would have longer and stronger thrust than conventional rocket engines. (Specific impulse.) SUMMARY: While chemical rockets combine chemical reactants (such as hydrogen and oxygen) for a quick burn, VASIMR exhaust gets much hotter then chemical reactants without burning. It heats a gas until it becomes plasma; the hotter the exhaust, the faster the rocket.
Momentum Exchange Equation m × v = M × V Momentum is mass times velocity; in a closed system, momentum exchanges are equal. EXAMPLE:  a space borne vessel is a closed system. Left Side Momentum equals Right Side Momentum mExh × vExh = MShip × VShip LEFT SIDE: vehicle emits tiny mass   × very high exhaust velocities. RIGHT SIDE: ship's larger mass × a small increase in ship's velocity. IMPULSE: Momentum per time.To determine impulse, divide each side by one second. mExh×vExh 1 sec = MShip×VShip 1 sec Redistribute terms as shown. mExh 1 sec × vExh = MShip × VShip 1 sec Define New Terms ---ffSec (fuel flow consumed per sec) ≈ exhaust mass per sec. ---a (acceleration) = ship velocity increase per second ffSec × vExh = MShip × a For simplicity, artificially assume: ---negligible mass growth due to relativity ---100% efficiency (all consumed particles contribute to thrust due to exhaust).	Initially, a traditional chemical rocket will insert the VASIMR vehicle into Earth orbit. Eventually, humans will enter this plasma powered vehicle for travel to interplanetary destinations. BACKGROUND:  Traditional chemical rockets are slow.  Chemical propulsion uses short duration "burns" to enter/exit transfer orbits. Such orbits are largely constrained by Kepler's laws of orbital motion around the Sun. VASIMR vessels are quicker than chemical rockets.  VASIMR's plasma engine stays on throughout the entire trip; thus, velocity constantly increases. In fact, it increases so much that flight profile calls for a "slow down" at mid-way. At exactly mid-way, vessel flips around to propel in opposite direction and start decelerating. Otherwise, the vessel would arrive at destination way too fast for orbital insertion around that planet.
Way too hot for material containers (exceeds 1,000,000°C); HOWEVER, plasma's charged ions respond very well to magnetic forces. Thus, VASIMR uses a magnetic "flux tube",  an invisible sleeve shaped like a nozzle; a magnetic nozzle. Technology of plasma containment originates  from research in magnetic fusion, Dr. Chang-Diaz's original field. For magnetic fusion, keep plasma from physical walls;  (causes "sputtering," to degrade both walls and plasma) then, bring it to thermonuclear temperatures for plasma ignition. A persistent obstacle to fusion energy has been  the difficulty of containing hot, electrically charged plasma.  Ironically, this challenge presents an opportunity;  use a controlled plasma leak to propel spacecraft.	Given a fusion propulsion system, assume exhaust particle velocity of 1 million m/sec. ffSec = MShip×(a×sec) vExh ffSec = .5×106gm×5×10-3m/sec 106m/sec ffSec = .025 m Assume exhaust particle vel:  vExh = 106m/sec Assume ship size:  MShip= 5 mTs = 5×106 gm Assume ship acceleration:  a ≈ 5×10-3 m/sec2 ffDay = .025gm sec × 86,400 sec ffDay = 2.160 kg ffDay = .00216 mT Thus, a particle exhaust rate of .025 gram/sec will propel the 5 metric Tonne (mT) vessel 5 millimeters/sec faster for each second of powered flight. .025 gm is a small quantity of mass; how much fuel would be required for constant thrust throughout the 90 day voyage to Mars? First, compute vessel's daily fuel requirement. If a 5 metric Tonne (mT) vessel expends .025 gm of plasma for every second of powered flight, then daily fuel consumption is .00216 metric Tonne. %TOGW ≈ t2-way× ffDay MShip = 180day× .00216mT 5 mT×day =  7.776% Fuel requirement for entire voyage can be computed as a percentage of ship's initial gross weight.
Given above flight profile,  determine what size ship  can VASIMR propel  with fuel consumption rate  of one gram per second? Assume exhaust particle velocity: vExh = 106m/sec Assume ship acceleration: a ≈ 5×10-3m/sec2 Assume fuel consumption:  ffSec = 1 gm/sec MShip = ffSec × vExh (a×sec) MShip = 1 gm × 106m/sec 5×10-3m/sec = 2×108 gm MShip = 200mT For every gram of ffSec, MShip equals 200 mT. MShip = ffSec × 2x108 × 1 mT 106gm = 200 ffSec mT	.
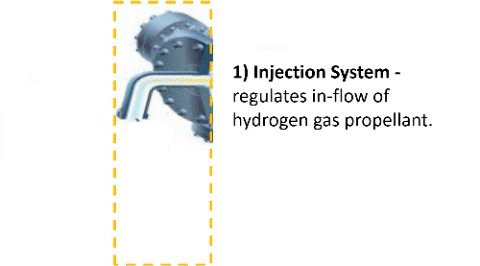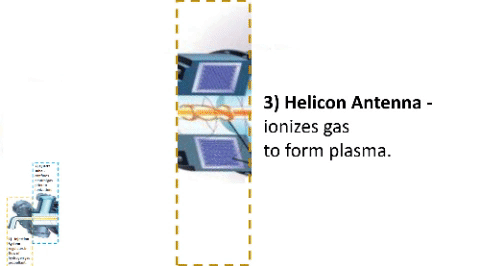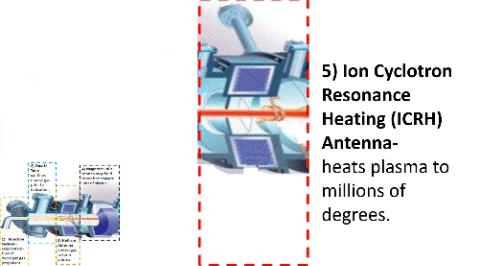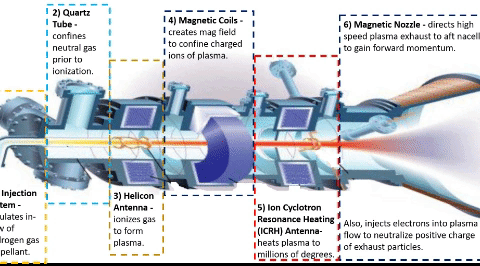Thus, TE considers Three Ways of Traveling to Mars:
1) Current Technology - Chemical Reactants: Use short burn to leave Earth's orbit, enter a transfer orbit from Earth's orbit to Mars's orbit; then, accomplish a 2nd short burn to enter orbit around Mars. With only chemical reactants, the shortest possible trip would be 6 months in a partial orbit. Most trips call for a much longer duration. Most practical use for chemical reactants would be to move vehicle from Earth's surface to Low Earth Orbit (LEO).  Even this dangerous maneuver could be avoided once a space elevator is constructed.	2) Pending Technology - Plasma Drive: Use plasma leaks for constant (though small) thrust throughout the trip. This propulsion provides a constant acceleration to midway; then, deceleration from midway to destination.  The resulting trip could be as short as 3 months.   While shorter than 6 months, 3 months is a long time to spend in near 0-g conditions. A more practical use for a plasma drive engine (such as the VASIMR) might be as a plasma injector into a particle accelerator propulsion system.
VASIMR Diagram High temps (exceed 1,000,000°C) greatly expand fuel’s volume as it transforms from gas to plasma. However, relativistic effects are slight as mass increase is at most one thousandth.
3) Thought Experiment:  Particle Accelerator Propulsion Thought Experiment (TE) suggests a third choice. Present day accelerators routinely take ions to near light speeds; thus, TE conservatively assumes exhaust particle velocity of .866c (approaching light speed) which introduces a relativistic growth factor (n) of 2 (particle goes so fast, mass doubles). Considering both this enormous velocity and relativistic growth of exhaust particles, TE uses simple momentum exchange equation to show that particle exhaust flow of .1 gm/sec will propel a 5 mT vehicle about 10 m/sec faster for every second of powered flight. Such acceleration provides g-force to simulate Earth surface gravity (g-force) during powered portions of flight. Furthermore, a trip time to Mars reduces from months to just days, a much more reasonable duration.	MShip × g = ffExh× VExh MShip = n × ffSec × .866c g × sec MShip = 2×.1gm×(259.1×106m/sec) 9.80665 m/sec MShip = 5.29×106 gm ≈ 5.3mT Simple momentum exchange equation shows fuel consumption of .1 gm/sec which grows to particle exhaust flow of .2 gm/sec which propels a 5 mT vehicle about 9.8 m/sec faster for every second of powered flight.
1. Exhaust Particle Velocity (VExh) Exhaust speed of particles expressed as decimal light speed VExh = dc × c dc, decimal component of light speed, can be computed:   dc = vExh /c  If vExh is already expressed as decimal c,  then determine dc by inspection. (Example: if vExh = .866c; then,  dc = .866.)	TYPE DRIVE VExh REMARKS Chemical Reactants 0.0c If no "burn" during entire trip, then exhaust particles are static inside the vehicle. Plasma  Drive .0033c TE generously assumes controlled plasma leak at 1 million m/sec, about 1/3 of 1% light speed (c). Particle Accelerator .866c TE conservatively estimates exit speed from particle accelerator at 86.6% c; much less then routinely achieved with current technology.
TYPE DRIVE AShip REMARKS Chemical Reactants 0 g With no propulsive force, ship is constrained to orbital velocity. Thus, TE assumes zero acceleration. Plasma Drive .0005g  AShip = 19.2845 km/sec  45 days 1) Reduces to average velocity gain  of about 0.005 m/sec.  2) Divide by g (9.8m/sec²) to convert to "decimal g".  3) Result: Less than 0.1% Earth gravity, very little g-force! Particle Accelerator 1.0 g g is 9.80665 m/sec², acceleration of a free falling object near Earth's surface. TE assumes g as the value for ship's acceleration.  EQUIVALENCE:  Crew feels same downward force (g-force) as Earth surface gravity. AShip: Acceleration of vessel is in decimal g.	2. Ship Acceleration (AShip) EXAMPLE: Typical plasma drive for typical Mars profile. AShip = ΔV  Δt = (V1 - V0) (t1 - t0) Let velocity: V0 = 0; let time: t0 = 0. Let V1 = VMax; let t1 = tMid. Step 1: Determine acceleration in meters per second per second (m/sec2).  AShip = VMax  tMid = 19.2845 km/sec 45 days AShip = 19,284.5 m/sec 3,888,000 sec = .00496 meter sec²  Step 2:    Convert to decimal g (dg) as shown. dg = AShip g = .00496 m/s² 9.8065 m/s² = .0005 g
3. Ship Mass (MShip) G-force Examples ffSec Plasma Drive MShip Particle Accel MShip 1 g 201.77 mT 26.47 mT 2 g 403.52 mT 52.94 mT 3 g 605.29 mT 79.42 mT Given dc dg ×  c g × ffSec dc ×  c g × ffSec dc= .0033; dg= .0005 Let dc= .866; dg= 1 NOTE: Ignore relativistic growth in this example. Later, we point out rate of fuel consumption (ffSec) differs from the rate of fuel particle exhaust (ffExh). c = 299,792,458 m/s g = 9.8065 m/s per second Thus, for each second, constant: c/g = 30.57×106  If ffsec  is in grams (kg), MShip is in metric Tonnes (mT). MShip = (dc×c) × (ffExh)  / (dg×g)	TYPE DRIVE MShip REMARKS Chemical Reactants n/a With no propulsive force, ship's mass is unconstrained. Plasma  Drive 201.7×106ffSec Given particle exhaust speed at 1,000,000 m/sec, and ship acceleration about .0005g, ship's mass is constrained at about 200 million times the mass of exhaust fuel expelled per any given second. MShip = dc dg × c g × ffSec = 2.017×106 ffSec Particle Accelerator 26.47×106ffSec Ship's weight is constrained to about 26 million times the mass of exhaust fuel expelled per second.(disregard relativity) CONCLUSION For one gram/sec (ffSec) of consumed plasma ions,  plasma drive might potentially push a heavier load at a much slower acceleration than Part. Accel. HOWEVER, total travel time is much longer duration.  (For Mars, plasma drive trip might take 90 days  vs. 2 days for Particle Accelerator.) TE assumes typical plasma drive vessel could not  maintain this fuel consumption rate for so long.
Two kilograms/second of exhaust particles at .866c can g-force propel a vehicle of 52 kiloTonnes. However, g-force fuel consumption must factor in Relativity and Efficiency.
TYPE DRIVE n REMARKS Chemical Reactants 1 Since static particles have zero velocity, relativity does not bear. Plasma  Drive 1.001 Since one million m/sec is slow relative to c, relativistic mass growth is very slight. Here, it is optimistically estimated as a thousandth. Particle Accelerator 2.000 At .866c, Lorentz Transform tells us that particle's relativistic mass is double the particle's mass at relative rest.	4. Growth Factor (n) Relativistic Growth (n) factor comes from the Lorentz Transform which can be used to quantify mass growth due to relativity. *** ffSec: fuel flow per second, mass per second consumed at rest.  This quantity converts to plasma prior to injection into particle accelerator. Note: n can be any rational number >1.0, but choosing certain dc values gives integer growth factors.  Choosing particle exhaust speeds with corresponding growth factors as integers is a convenience but in no way a requirement.
n = 1 √(1-dc2) dc= √(n2-1) n √(n2-1) From Lorentz  Transform (LT) Solve LT for dc Product of dc × n. n dc  1 0.0 c 2 .866 c 3 .943 c Given √(n2-1) n ffExh: exhaust mass per second exits the spacecraft for propulsion. Due to relativistic speeds via particle accelerator, consumed fuel mass grows to become fuel flow exhaust. ffExh =  ffSec √(1-dc2)  =  n × ffSec

5. Efficiency (E)... ..., a value between 0 and 100%, which quantifies how much input is deflected from output performance.  This decrease reflects inevitable design flaws and peripheral needs.  Theoretically, a perfect design would yield: E= Input/Output = 100%. Alas, perfect efficiency will always elude us; however, continuing design improvements will always  take us ever closer.  Thus, propulsion efficiency will likely start out low as we journey to nearby destinations (like Mars and Ceres). However, TE assumes E will gradually increase as we design better vessels to journey to further destinations.	Relativity: as initial fuel mass speeds up from relative rest (0 m/s) to .866c, initial 1.0 kgm per sec grows to an exhaust flow of 2.0 kgm per second. Theoretically, this should g-force propel a vehicle of 53,000 mTs. MShip = (dc×c) × (n×ffsec)  / g MShip = dc × 30.57×106× (n×ffsec)  MShip = .866 × 30.57×106× (2×1.0 kg) = 52,947 mT  However, above equations disregards inevitable inefficiencies.  Like all other systems, G-force spaceships will never achieve 100% efficiency; however, the conversion rate from at rest, consumed particles (ffsec) to near light speed, exhaust particles (ffExh) will improve over time.
TE previously disregarded efficiency concerns to determine a g-force ship of 52,666 mTs theoretically requires consumption rate of 1.0 kg/sec (ffSec).  This consumed quantity of particles must accelerate to .866c (VExh) to relativistically grow to 2.0 kg of exhaust particles (ffExh). Since inefficiency is inevitable, optimistically assume E=70%. thus, we assume 70% of the 2.0 kg survives the particle acceleration process.  Sadly, this results in 70% of the desire thrust with only 70% g-force.  G-force vessel must compensate for this shortfall by increasing the consumption rate.	Since system inefficiencies are inevitable, actual thrust produced from theoretical fuel consumption requirement will be much less then needed for g-force. For this example, TE optimistically assumes system efficiency of 70%.  Thus, system inefficiencies divert 30% of plasma particles away from the thrust stream. Relativity increases the consumed 1 kg of fuel to 2 kg; HOWEVER, only 1.43 kg would actually exit the vehicle in the exhaust flow. In this arbitrary scenario, an on-board weight scale would measure a 100 lb object at only 70 lbs. CONCLUSION: At 70% efficiency, fuel consumption of 1.0 kg produces enough thrust for only .7g-force. Thus, TE uses an efficiency factor (ε) to determine added consumption required for g-force.
Efficiency Factor (ε) is reciprocal of Efficiency (E). ε = 1/E To compensate for inevitable inefficiencies, consumption rate must increase. Therefore, determine the Efficiency Factor (ε) by dividing 1 by E.  Then, determine increased consumption rate by multiplying original rate by ε. EXAMPLE:  if E is 70%, increase consumption from 1.0 kg/sec to 1.43 kg/sec as shown in diagram. TE now uses arbitrary examples of E = 70% and ε = 1.43 as a starting point. Actual g-force flights will provide empirical data tp determine actual Efficiency (E) and Efficiency Factor (ε). Items contributing to inefficiencies might include: Design flaws, for example, particle collisions will likely stop a significant portion of particles from exiting vessel.  Arbitrarily assume this causes 10% inefficiency. Systemic power needs; propulsion system components will need power. particle accelerator devices such as collision detectors, possible even the magnets (see next chapter for more on magnets) will need power even though these components don't directly contribute to propulsion. Arbitrarily assume this causes 10% inefficiency. Auxiliary Power. Life support, comm gear, nav gear and many other items are needed for ship operation and crew comfort, but not part of propulsion system. However, a portion of propulsion power will likely be diverted for these purposes. Arbitrarily assume this causes 10% inefficiency. E  = 100% - 10% -10% -10% = 70% Actual efficiency data can't be obtained until g-force operations actually begins. Thus, TE must make do with assumptions.  For above three items, TE assumes each requires 10% of propulsion power output: thus, TE arbitrarily assumes an efficiency of 70% (a very optimistic assumption.)  Actual efficiency (E) will need to be validated with empirical data.  ffSec =  ε × MShip  / (dc × 30.57×106 × n)  ffSec  = 1.43 × 52,947 mT / (.866 × 30.57×106× 2 × 1.0) = 1.43 kg	TYPE DRIVE E REMARKS Chemical Reactants 100% Perfect efficiency because no fuel expended, a tautology. Plasma  Drive 100% Very little fuel expended per day; so, this efficiency is close to one. Particle Accelerator 70% Considerable fuel consumption; thus, inefficiencies are inevitable.  If exact values are not yet known, make reasonable assumptions. For g-force propulsion, a 53 megaton vessel must emit an exhaust flow (ffExh) of 2 kg/sec with particle velocity of .866c. To achieve this exhaust flow, theoretical "at rest" fuel consumption rate is 1.00 kg/sec; and relativity will grow the particles to 2.0 kg/sec.  HOWEVER, practical fuel consumption must consider inevitable inefficiencies. Thus, apply the Efficiency Factor (ε), the reciprocal of Efficiency (E).   EXAMPLE:  For E=70%, ε = 1/E = 1.43.  Increasing 1 kg by a factor of 1.43 results in 1.43 kg/sec consumed at rest.  Accelerate this to .866c, and it relativistically grows to 2.86 kg. Since this example assumes 70% survival rate, the exhaust particle flow reduces to 2.0 kg, the amount needed to create g-force.
6. Daily Difference (∇Day) Ship's mass decreases due to powered flight's fuel consumption.  TE uses term, ∇Day , to describe daily decrease of ship's mass due to fuel consumption.  Recall that g-force ship capacity depends on actual quantity of high speed fuel particles (ffExh) actually expelled. Furthermore, g-force thrust particles are at relativistic velocities near light speed, dc c. Consider following factors: (1) Relativistic growth factor, n, depends on exhaust velocity (VExh). VExh = dc c  Compute n from Lorentz Transform (LT). n = 1 √(1-dc2) ffExh= n×fSec (2) Theoretical Exhaust Flow, ffExh As consumed fuel flow (ffSec) accelerates, mass grows by relativity growth factor, n. ffSec = ffExh n ε  = 1 E (3) Practical fuel consumption, ∇Sec. Fuel flow, ffsec, is consumed at relative rest.  ∇Sec  = ε×ffSec However, this fuel consumption must also account for inevitable inefficiencies. (Efficiency factor, ε, is reciprocal of Efficiency, E). ffDay = 86,400×∇sec (4) Daily fuel consumption can be approximated. Multiply by 86,400, seconds per day. (5) Recall previous work: c/g = 30.57×106 dc× n = √(n2 - 1) For a g-force spaceship, dg = 1. For other drive types, dg << 1. MShip = vExh×ffExh AShip = dcc×nffsec dgg = 30.57×√(n2-1)×106×ffsec dg	TYPE DRIVE ∇Day REMARKS Chemical Reactants 0 No fuel expended. However, no g-force during the flight, and no hurry to get there. Tolerable for robots but not humans. Plasma Drive 0.014% dg=.0005 ε=1 n=1.001 For current plasma propulsion, compute and apply very small values of dg. Particle Accelerator 0.233% dg=1 ε=1.43 n=2 Relativistic growth of n=2 due to exhaust velocity of .866 c, light speed. Resulting momentum enables g-force; thus, dg=1. Arbitrarily assume E = 70%  for  efficiency factor of ε = 1/E = 1/.7 = 1.43. Most daily fuel consumption,  requires .233% of vessel's GW. G-force benefits:  1) inflight comfort 2) quick flight time. (6) ∇Day is a percentage of ship's current gross weight (%GW). Daily decrease of ship's GW (∇Day ) depends on Efficiency Factor (ε), relativistic growth (n) and ship's acceleration (dg, expressed as decimal).  ∇Day = ffDay MShip ∇Day = ε× 86,400 × ffsec 30.57×√(n2-1)×106×ffsec/dg ∇Day = ε × dg × 86,400 30.57 × 106 × √(n2-1) ∇Day = ε × dg × 0.283% GW √(n² - 1)

7. Typical Flight Duration (t) Type Drive Travel TIme REMARKS Chemical Reactants 180 days Flight profile calls for a partial orbit at orbital speeds. Plasma  Drive 90 days A small amount of constant propulsion can reduce trip time by as much as half. Particle Accelerator 2 days A constant amount of near light speed propulsion particles can reduce trip time to much shorter durations in much more tolerable conditions

8. TOTAL DIFFERENCE (∇Ttl) To determine trip's total fuel consumption, accummulate all the daily GW differences for entire trip. NOTE: ∇Ttl = GWFinal - GWInitial= Σ∇Day  Quick way to approximate: Multiply daily diff (∇Day) times total trip time (t). ∇Ttl = ∇Day × t This works well for extremely small daily fuel consumptions or for short trip durations. Both these circumstances exist in following table. TYPE DRIVE FUEL REMARKS. Chemical Reactants 0 No fuel expended during flight. Disregard chemical reactions ("burns") to enter/exit orbit of flight. Plasma  Drive 1.26% For extremely small daily fuel consumptions,  product of trip time times daily fuel consumption: t × ∇Day = 90 dy × .014%GW/day  t × ∇Day  ≈ 1.26% GW0 Particle Accelerator 0.466% For a short 2 day trip, approximate as follows: t × ∇Day = 2 dy ×.233% GW/day  t × ∇Day ≈ 0.466% GW0

EXPONENTIALS However, many flight profiles have significant fuel consumption and/or lengthy trip duration.  EXAMPLE: Let daily fuel consumption to be 1% ship's gross weight (GW). ∇Day = 1% GW/day = .01 GW/day EXAMPLE:  Let total trip duration (t) be 40 days. To determine total fuel consumption, our intuition might mislead us.  Initially, we might estimate total fuel consumption at forty times one percent, for a fuel burn of 40% initial gross weight (GW0).  Thus, if GW0 = 100 mTs; then, total fuel consumptions might be 40 mTs, and final gross weight (GW40) might be 60 mTs.  However, this intuitive approximation would be very imprecise. ∇Ttl = .01 GW/day × 40 days ≈  .4 GW0 GWFin =  GW40 ≈ GW0 - ∇Ttl = .6 GW0 Multiplying daily differences is easy but inaccurate!!! A more accurate model of fuel consumption  might involve exponents. Axiomatic: If daily fuel consumption is 1% gross weight (∇t = .01 GWt), then tomorrow's gross weight will be 99% of today's; GW1 =  .99 GW0 Example: If ship's initial gross weight GW0 is 100 metric Tonnes (mTs), then ship's GW can be computed for first, second and following days: Day 1: GW1 = .99 GW0 = 99 mT Day 2: GW2 = .99 GW1= .99 × 99 mT =  98.01 mT Day t: GWt = .99 GWt-1= (1 - ∇Day)t × GW0 Day 40: GW40 = .9940  × 100 mT =  66.897 mT Exponentials more accurately determine fuel consumption  for longer duration flights.

SUMMARY:  In coming days of powered flight, ship's Gross Weight (GW) decreases due to fuel consumption.  Subsequently, slightly lighter ship requires slightly less fuel; thus, absolute fuel consumption decreases even though percentage Gross Weight (%GW) remains the same.  To model this, TE initially considers a convenient rate of fuel consumption (ffsec=1.0 kg/sec); then, TE determines ship's GW. For particle exhaust speed (VExh) of 86.6% light speed (c),  relativity doubles mass size; thus, growth factor(n)=2. Thus, √(n2-1) = 1.73.  For g-force propulsion,  dg = 1.  MShip = 30.57×√(n2-1)×106×ffsec dg For convenience, consider fuel flow (ffsec) of 1.0 kgm/sec.  Theoretically g-force propels a ship of 52,886 mTs. However, it's much more likely that flight planners will first determine Take Off Gross Weight (TOGW); then, flight engineers will determine practical fuel flow requirements based on planned TOGW and known efficiency factor (ε). FIRST CONSIDERATION: Ship's Gross Weight (GW) requires specific fuel consumption.  Rearrange and adjust for best known efficiency factor (ε) to determine ∇sec. Let ε = 1.43, same arbitrary value as current example. Let MShip = 100,000 mTs. ∇sec = 2,697 gms / sec ∇sec = ε×ffsec = ε × MShip 30.57×1.73×106 2ND CONSIDERATION: Fuel consumption decreases ship's GW each day of powered flight. Ship size shrinks during powered flight due to fuel consumption.  Smaller GW means decreased fuel requirement; thus, fuel consumption decreases. ∇Day = 0.233% / day TOGW = GW0  = 100,000 mT  t %GWt GWt Ft ffsec days %TOGW metric Tonnes mT/day gm/sec 0 100.00% 100,000 mT  n/a n/a 1 99.77% 99,767 mT 233.00 2,697 2 99.53% 99,530 mT 232.46 2,690 ... . . . . . . . . . . . . 40 91.09% 91,090 mT 212.74 2,462 Given (1-ΔDay)t %GWt×TOGW GWt×ΔDay Ft 86,400 (NOTE: TE arbitrarily chooses to recompute GW daily.)  Define Gross Weight (GW) GW = Structure + Payload + Fuel While structure and payload remain fixed throughout the voyage, fuel decreases throughout powered flight. Assume first day's consumption is .233% of 100,000 mT or 233 mT;  then, compute GW for end of day 1: GW1 = GW0 - (GW0×∇Day) = 100,000mT -233 mT = 99,767 mT  Second day's fuel consumption could be computed as  .233% of first day's GW: F2= ∇Day × GW1  = .00233 × 99,767 mT = 232.46 mT Consider much longer flights. For an indeterminate flight duration, any given day's fuel consumption should use exponents as shown: Ft= ∇Day × GWt=  ∇Day × (1-∇Day)t× GW0 Example: 40 days. Someday, a g-force trip to Kuiper Belt could require 40 days. Thus, reconsider previous example of 40 days duration with ship size of 100 kiloTonnes.  F40= .00233 × (.99767)40×100,000 mT =212.74 mT Decreased daily consumption translates into smaller fuel flow rates. To determine fuel flow for Day 1,  divide total fuel consumption by total seconds per day. ffsec = Ft / 86,400 sec = 233×106 gm/86,400sec ffsec = 2,696.8 gm/sec  Similarly, Day 2's 232.46 mTs translates into 2,690.5 gm/sec and so on for succeeding days.  For the last day (Day 40), we get 2,462.3 gm/sec,  a significant 234.5 gm/sec less then the initial flow fuel for Day 1.  Thus, to maintain constant g-force throughout powered flight, fuel flow must be constantly monitored and adjusted. G-force sensors (i.e., scales with 100 lbs. weights should indicate 100 lbs. throughout powered flight).  Sensor servo connection could automatically adjust fuel flow.

Sidebar: SPECIFIC IMPULSE(Isp) Type Drive VExh Isp Solid rocket 2,500 m/sec 250 sec Bipropellant liquid rocket 4,400 m/sec 450 sec Ion thruster 29,000 m/sec 3,000 sec VASIMR 30,000-120,000 m/sec 3,000-12,000 sec Part. Accel. Propulsion .866c≈260,000,000 m/sec 26,000,000 sec GIVEN OBSERVED VExh /g Specific impulse is measured in seconds. EXAMPLE: one pound of typical solid rocket motor fuel produces one pound of thrust for 250 seconds. Specific Impulse (Isp) is the length of time (usually “seconds”) that each unit weight of propellant propels its own weight.  For ships in vacuum of space, it proves convenient to compute specific impulse as average particle exhaust speed divided by g, near Earth gravity: Isp = VExh/g Isp is the specific impulse measured in seconds VExh is the exhaust speed along the axis of the engine in (m/sec) g is an object's free fall acceleration at the Earth's surface (approx. 10 m/sec2) In contrast, Impulse (I) is the average propulsion Force (F) times the total duration (t) of firing. I = F × t Straight forward work rearranges terms for Impulse equation such that Impulse equals exhaust mass flow times velocity of exhaust particles. F = ma = m × v/t I = F × t = m × v (i.e., momentum) For now, assume perfect efficiency; thus, exhaust fuel flow equals input fuel flow: I = ffExh × VExh Effects of relativity on size of exhaust particles. ffExh = n × ffsec For VASIMIR rocket engine, relativity is negligible and n=1 I = ffsec × vExh Recall ffExh is the amount of exhaust mass per time ejected out of the rocket (adjusted for relativity). Assuming constant exhaust velocity, we get: I = ffExh × vExh where m is the total mass of the propellant. We can divide this equation by the weight of the propellants to define the specific impulse. The word "specific" just means "divided by weight". Thus, Specific Impulse (Isp) shows Impulse from each unit weight of propellant. For example, typical solid rocket motor produces 250 pounds of thrust for every pound of fuel injected into the combustion chamber (per second). Expressed mathematically: Isp = F/ffsec  Specific Impulse (in seconds) = Isp  Thrust (in pounds) = F Fuel flow (in pounds/second) = ffsec  The specific impulse Isp is given by: Isp = Veq / g0 where g is the gravitational acceleration constant (9.8 m/sec2 in metric units).If we substitute for the equivalent velocity in terms of the thrust:  Isp = F / (ṁ × g0) Mathematically, the Isp is a ratio of the thrust produced to the weight flow of the propellants. A quick check of the units for Isp shows that: = (m/sec) / (m/sec2) = sec Why specific impulse? Use fuel flow (through the exhaust nozzle) to determine rocket thrust. It indicates engine efficiency. A higher value of specific impulse shows greater efficiency, more thrust for the same amount of propellant. It simplifies; Isp units are the same for English units or metric units. Preliminary analysis: It gives us an easy way to "size" an engine during The result of our thermodynamic analysis is a certain value of specific impulse. The rocket weight will define the required value of thrust. Dividing the thrust required by the specific impulse will tell us how much weight flow of propellants our engine must produce. This information determines the physical size of the engine. c/g = 30.57×106 m/s

CONCLUSION:  G-force vessel must vary daily fuel consumption.

Fortunately, VASIMR's first name is "Variable"; thus, will vary plasma quantity injected into particle accelerator.

SLIDESHOW

---

---

---

VOLUME O: ELEVATIONAL VOLUME I: ASTEROIDAL VOLUME II: INTERPLANETARY VOLUME III: INTERSTELLAR

---

---

---