SUPER G-FORCE

If g-force is great, is super g-force even greater?

---

Expectations continually adjust to ever higher levels. For example, it's very possible that someone will win one million dollars in a lottery; you'd think she'd be happy forever. However, relevant experience indicates such happiness lasts for a much shorter time.

---

Our neophyte millionairess will soon learn that a million dollars is not infinite, and materialistic desires will likely grow to accommodate that finite amount. For instance, she can now fly first class, but she can't yet buy a private jet.

---

In similar fashion, consistent g-force propulsion will prove a great boon to humankind due to very short travel times to nearby planets as well as inflight comfort from simulated Earth-like gravity. However, humans will soon want more stuff shipped even faster. Humans probably won't travel at greater than one g-force, but shipments of essential materials could be greatly expedited if they could fly at super-g forces.

---

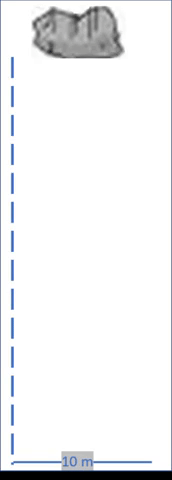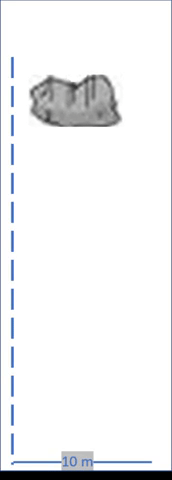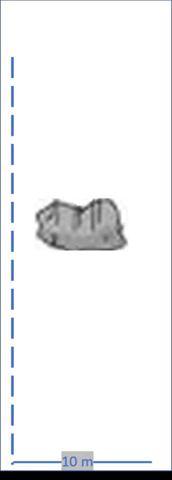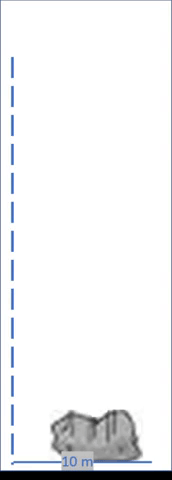In 1 second, 2-g moves falling rock to 20 m/s over 10 m.  In 1 second, 1-g moves falling rock to 10 m/s over 5 m.  Near Earth's surface, a free falling object will accelerate at rate g, approx. 10 m/sec2. Thus, an acceleration of 2 g would be 20 m/sec2. Using Newtonian motion equations, we've previously determined that first second of 1-g free fall will move object 5 meters. Extend  to one day of constant g-force acceleration to move vessel to .25 Astronomical Units (AUs).	Expand our thought experiment's envelope to exceed g-force acceleration for selected vessels. Assume no humans, but Artificial Intelligence (AI) devices will guide a vessel with essential cargo. Express 2g as different units of distance/time. 20 m sec2 ≈ 2g ≈ 20 m sec2 × (86,400 sec)2 day2 × 1 AU 1.5×1011m ≈ 2g ≈ 1.0 AU day2 If double g-force does not damage ship or contents; then, much shorter travel time would be an enormous benefit.   d = a×t2 2 ≈ 1.0 AU day2 × (1 day)2 2 = 0.5 AU let a = 2g = 1.0AU/day² Note that one second of 2-g free fall moves an object for 10 meters; thus, extend duration to one day (86,400 seconds) for distance of 0.5 AU.
Describe greater accelerations as integer (i) times g, free fall acceleration experienced near Earth's surface. DISTANCE EXAMPLE:  After one day of 2g-force acceleration, object travels a great distance: dAccel = .5 × a × t2 = .5 × 2g × t2 = .5 × 2(.5AU/day2) × day2 = .5 AU
ACCELERATE TO GREAT SPEEDS QUICKER! After one day (=24 hrs × 3,600 sec/hr =86,400 sec) of 2×g-force acceleration, VMid ≈ 2g × t ≈ 20 m sec2 × 86,400 sec = 1,728,000 m sec vessel increases velocity to 1,728 km/sec, an enormous speed! For current example, let this happen at midpoint. VMid ≈ 1,728 ,000 m sec × 1 km 1,000 m =  1,728 km sec Thus, vessel must SLOWDOWN during 2nd half of trip, which takes a day of 2g deceleration. During 2nd day, vessel's speed decreases at 2g to 0 km/sec. VDest ≈ VMid - 2g × t  ≈ 1,728,000  m sec - 20 m sec2 × 86,400 sec RESTATE: Accelerate  at negative rate (-2×g) for 2nd day VDest ≈ 1,728 km sec - 1,728 km/sec day × 1 day =  0 km sec to return to initial velocity.	QUICKLY DECELERATE TO ORBITAL SPEED! AXIOMATIC:  2nd day deceleration distance from midway will equal first day’s acceleration distance to midway: dDecel ≈ .5×-a×t2= .5×-2g×t2  ≈ .5 × -2 .5AU day2 × 1day2 = -.5AU ABSOLUTE DISTANCE: [dDecel] = .5 AU = dAccel Thus, the sum of acceleration distance (for first half of voyage) and deceleration distance (for final half) is the total distance; or total could be restated as twice the acceleration distance.  dAccel + [dDecel ]= dTtl = 2 (.5×a×t2) Determine total distance for a 2-g vessel to accelerate for one day and decelerate for 2nd day. dTtl ≈ 2(.5×2g×t2) ≈ 2 [.5×2× .5AU day2 ×1day2] = 1.0AU Both G-force and super G-force  vessels require following:  1) Acceleration to midway for first half of trip.  2) Equal but opposite propulsive force for 2nd half.  Thus, vessel attains useful, orbital speed at destination.
Distances: Different G-forces One Day Accelerate; 2nd Day Decelerate Acceleration (a) Velocity (V= a×t) Distance (a×t2/2) Same value, different units. After one day's acceleration. dAccel dDecel dTtl i × g m/sec2 AU/day2 km/sec AU/day % c AU AU AU 1 g 10 0.5 864 0.5 0.288%c 0.25 0.25 0.5 2 g 20 1.0 1,728 1.0 0.576%c 0.50 0.50 1.0 3 g 30 1.5 2,592 1.5 0.864%c 0.75 0.75 1.5 Given i × 10 m sec2 i × .5 AU day2 i×(864km/s)×t day i×(.5AU)×t day2 i×(.288%c)×t day i×(.5AU)t2 2×day2 = dAccel = dAccel + dDecel gravity force (g): experienced by all who reside on Earth's surface.   10 m/sec²: acceleration experienced by freely falling objects near Earth's surface.   0.5 AU/day²: transform 10 m/sec² into units more convenient for g-force, space travel. km/sec: 1 g speed increases 10 m/sec for every sec of entire day (86,400×10 = 864 km/sec); an enormous speed.   AU/day: for a more convenient measure, transform 864 km/sec to 0.5 AU/Day.   %c: 1 day of g-force achieves small portion of light speed (c). 864 km/sec ÷ c = .288% dAccel: Acceleration distance   dDecel: Decel. dist. = Accel dist.   dTtl: dAccel + dDecel = 2×dAccel Determine distance after one day's acceleration followed by one day's deceleration.
For a given set of Super-G's determine flight time for a given distance, d.  tAccel = √(d/a), estimates acceleration time to halfway. EXAMPLE: Typical distance from Earth to Jupiter: d = 5AU. dAccel = dDecel = d/2 = 2.5 AU tAccel = √ 2 × dAccel a = √ d 3g = √ 5 AU 1.5AU/day2 = 1.826 days let a = 3g = 1.5AU/day² tAccel + tDecel = t = 2 × tAccel = 2√(d/(3g))= 3.652 days	Accelerate to midway for half of distance. Decelerate to destination for remaining half. Travel Times for Different G-forces Acceleration (i ×g) 1 g 2 g 3 g Dest Typical  Distance Flight Time NEO 1 AU 2.8 day 2.0 day 1.6 day Mars 2AU 4.0 day 2.8 day 2.3 day Jupiter 5AU 6.3 day 4.5 day 3.7 day Given Observed 2 √ d i × .5AU/day²
Total time considers acceleration to halfway: √(d/a) plus equal time for deceleration.
Fuel Consumptions: Different G-forces Both acceleration and deceleration require powered flight, which requires fuel.
Assume for interplanetary flights, particle exhaust speed, VExh  = .866 c Thus, decimal component of light speed: dc = VExh /c = .866 From Lorentz Transform, growth factor: n = 1/√(1-dc2)  = 1/√(1-.8662) = 2 At  particle velocity of 86.6%c, exhaust flow is twice fuel consumption at rest: ffExh = 2 × ffsec Previous work leads to following equation: ∇ = i × g × day c × √(n2-1) = i × .288% √3 = i × .1663% "i" can be a single digit integer (i.e., 1,2, 3, ...) as shown below. Fuel Consumptions for Different G-forces Acceleration (n×g) 1 g 2 g 3 g ∇=.17%TOGW ∇=.33%TOGW ∇=.50%TOGW Dest Typical  Distance Flight Time Corresponding Fuel Consumed NEO 1 AU 2.8 day 2.0 day 1.6 day 0.94%TOGW 1.32%TOGW 1.69%TOGW Mars 2AU 4.0 day 2.8 day 2.3 day 1.32%TOGW 1.88%TOGW 2.30%TOGW Jupiter 5AU 6.3 day 4.5 day 3.7 day 2.10%TOGW 2.98%TOGW 3.70%TOGW Given Observed 2 √ d i×.5AU/day² Fuel: f = t × ε ×∇ Efficiency Factor (ε) arbitrarily chosen as 2.0 for interplanetary travel.	Define Variables: 1) ∇, daily difference = daily percentage of ship's mass needed to convert to exhaust particles to propel ship in opposite direction. 2) g, from expression "g-force", g is acceleration due to Earth surface gravity. For convenience, round g's value to 10 m/sec2. 3) day = quantity of seconds per day = 86,400 sec. 4) c, light speed. Round to 3×108m/sec. 5) Given above approximations, g×day/c = .00288 = .288%. 6) n, growth factor, is the amount of relativistic mass increase due to high speeds achieved by accelerated particles. Thus, exhaust fuel flow, ffExh is greater then ffsec , original fuel flow per second: ffExh= n × ffsec ffExh = ffsec √(1-dc2) n = 1 √(1-dc2) 7) dc is decimal component of VExh, exhaust particle speed expressed as decimal light speed. Thus, dc =  VExh c While particle exhaust velocity (VExh) can range from zero to just short of c, assume interplanetary voyages use a reasonable value: vExh= 86.6% c = .866 c.

Operational Flight Plan Different Super-G's

Determine time of flight after halfway acceleration followed by halfway deceleration.

For even faster interplanetary transport, consider super G-forces even greater than 3g.
With acceleration as i × g, "i" values could include both integers and decimals.

		Let VExh = .866c					∇= i × .1663%
		a = 1 × g		a = 2 × g		a = 3 × g		a = 4 × g		a = 5.5 × g
		∇=0.17%		∇=0.34%		∇=0.50%		∇=0.67%		∇=0.91%
Dest	Dist. (d)	Time(t)	Fuel (f)	Time	Fuel	Time	Fuel	Time	Fuel	Time	Fuel
	Typical	days	%TOGW	days	%TOGW	days	%TOGW	days	%TOGW	days	%TOGW
NEO	1 AU	2.83	0.94%	2.00	1.33%	1.63	1.62%	1.41	1.88%	1.31	2.20%
Mars	2 AU	4.00	1.32%	2.83	1.87%	2.31	2.29%	2.00	2.64%	1.71	3.10%
Jupiter	5 AU	6.32	2.09%	4.47	2.94%	3.65	3.60%	3.16	4.15%	2.70	4.86%
Saturn	10 AU	8.94	2.94%	6.32	4.13%	5.16	5.05%	4.47	5.81%	3.81	6.80%
Uranus	20 AU	12.65	4.13%	8.94	5.79%	7.30	7.06%	6.32	8.12%	5.39	9.49%
Neptune	30 AU	15.49	5.03%	10.95	7.05%	8.94	8.58%	7.75	9.85%	6.61	11.48%
Kuiper Belt	40 AU	17.89	5.79%	12.65	8.10%	10.33	9.84%	8.94	11.29%	7.63	13.14%
Given	Observed	t = 2 √ d i×.5AU/day²					f=100%-(1-ε×∇)t

100 AU from Sol

This is much further than the Kuiper Belt (KB), but only a miniscule portion of distance from Sol to the edge of the Solar System.

NOTE:  Many people used to consider the KB to be the "edge" of the Solar System, due to no planets detected beyond this belt of asteroids and comets.  We now know of planets well past the KB.

In any event,  Sol's influence extends well beyond the KB to the Oort Cloud (OC) with billions of comets.  

OC is about 1 LY in radius (about 65,000 AU) about 1/4 of distance to nearest stellar neighbor, Alpha Centauri (AC) Stellar system.

SCENARIO:  Normal passenger travel typically takes about 28 days to travel from Earth to this site at 1 g. Flight requires fuel of about 9% of vessels TOGW.  However, non-passenger transport could travel at higher g's to expedite transport of essential cargo.

WHY ORBIT AT 100 AU? Why deploy a habitat to where there are no planets, no moons, perhaps a rare glimpse of an asteroid/comet?

ANSWER:  At 100 AU, this habitat could become an extremely valuable resource; it can store and forward large volumes of communications from the inner solar system to ships heading farther out, eventually to the stars.

Quick look at following table tells us that a 4 g vessel can make same trip  in half the time of an one g flight while consuming twice the fuel

	a = 1 × g ∇=0.17%		a = 2 × g ∇=0.33%		a = 3 × g ∇=0.50%		a = 4 × g ∇=0.67%
Dist	time	fuel	time	fuel	time	fuel	time	fuel
(AU)	(days)	(%TOGW)	(days)	(%TOGW)	(days)	(%TOGW)	(days)	(%TOGW)
1	2.83	0.94%	2.00	1.33%	1.63	1.62%	1.41	1.88%
100	28.28	8.99%	20.00	12.50%	16.33	15.11%	14.14	17.26%

CONCLUSION: Interplanetary flight profiles for both g-force and super g-force propulsions
can be closely approximated with Newtonian motion equations.

SLIDESHOW

---

---

---

---

VOLUME 0: ELEVATIONAL
VOLUME I: ASTEROIDAL
VOLUME II: INTERPLANETARY
VOLUME III: INTERSTELLAR

---

---

---

---