EXAMPLE: Let particle exhaust speed = 56.5% light speed; then, dc = .565, and daily difference (∇) = 0.5% GW to produce g-force acceleration. For practicality, TE arbitrarily assumes inevitable inefficiencies increase actual consumption rate to twice daily diff (2×∇=1% GW) to cover design flaws and peripheral power needs.
REQUIRED FUEL
f = 1 - (1-2∇)t
|
RANGE
| R = | log(1-%TOGW)
log(1-2∇) |
|---|
|
 1) NEWTONIAN. G-force time for given distance to destination (d): t = √(2×d/g)
2) ACCELERATE to halfway (d/2). Determine g-force time: t = √(2×(d/2)/g) = √(d/g)
3) PLUS DECELERATE to destination, Double time from 2) : t = 2√(d/g)
4) PLUS RETURN. Double time from 3) . Total 2 way Travel time: t = 4√(d/g)
|
1% Solution to the Planets
Interplanetary G-force solution: 2∇ = 1% GW/day
Daily GW remainder is: (1-2∇) = 1 - .01 = 99%.
Thus, every day's GW is 99% of previous day's GW.
Determine fuel consumed:
initial GW (100%) minus expected GW(.99t).
Destination
|
Typical
distance (d)
|
Travel
time (t)
|
Required
fuel (f)
|
Jupiter
|
6 AU
|
13.9 day
|
13.0% TOGW
|
Saturn
|
10 AU
|
17.9 day
|
16.5% TOGW
|
Uranus
|
20 AU
|
25.3 day
|
22.5% TOGW
|
Neptune
|
30 AU
|
31.0 day
|
26.8% TOGW
|
Kuiper Belt
|
40 AU
|
35.8 day
|
30.2% TOGW
|
| Observed | 4×√d
√.5 AU/day² | 1 - .99t |
|---|
Range: R = log(1-%TOGW)/log(1-2∇)
R = log(1-.5)/log(1-.01) = 68.97 days
1% consumption rate
leaves plenty of margin
for two way
interplanetary destinations;
however, ...
|
| ...interstellar destinations need much better performance. |
|---|
 As particles go faster, mass increases.
Relativistic particles grow greatly.
Thus, exhaust fuel flow (fExh) exceeds consumed fuel flow (ffsec).
Restate ffsecwith n, mass multiple: ffExh = n × ffsec
Lorentz Transform (LT) quantifies relativistic mass increase of high speed particles. EXAMPLE: Let one second of consumed fuel flow, ffsec, = 1 gm; let it achieve a relativistic velocity, dc×c =.700c = 70.0% light speed prior to exhaust;
| n |
=
| 1
√(1 - dc2) |
=
| 1
√(1 - .7002) | = | 1.4 |
then, 1.0 gm of ffsec will become 1.4 gm of exhaust fuel flow, ffExh,just before it exits vessel and contributes to forward momentum.
Thus, slightly change ship's mass equation:
| MShip = | n × ffSec × | dc× c
g | = | dc
√(1 - dc2) | × 30.57×106 ffSec |
|---|
However, TE still assumes daily consumption is
because fuel is consumed at rest.
Thus, re-accomplish daily diff. equation:
| ∇= | ffDay
MShip | = | 86,400sec×ffSec
ffSec×dc×c/(g×√(1-dc2)) | = | 86,400 sec × g/c
dc/√(1-dc2) | = | .2826% GW
n × dc |
|---|
EXAMPLE: Let particle's exhaust speed (VExh) be .7c; then, light speed's decimal component, dc , is .7; mass multiple, n, is 1.4; and ship's gross weight daily difference, 2∇, computes as 0.58% GW. Subsequently, compute the daily gross weight remainder (100%- 2∇)= (1-.0058) = .9942.
During powered flight, the g-force vessel's GW is ever decreasing at the daily rate of 2∇; thus, the quantity, 1-2∇, is a recurring remainder, and the exponential, t, an compute ship's gross weight (GWt) after t days of powered flight. EXAMPLE; Let accel time (t) be 30 days.
GWt = GW0(1-2∇)t= (1-.0058)30 GW0 = 84.1% GW0
If t, acceleration time, is known (Example: t = 60 days) ; then, determine fuel required:
f t= GW0(1-(1-2∇)t) = (1-(.9942)30)GW0 = 15.9% TOGW
Under above conditions, vessel must expend 15.9% of initial GW to maintain g-force for 30 days (NOTE: Artificially estimates efficiency as 50%.)
|
| Relativity Increases G-force Effect |
| ffExh × VExh / g = MShip = n × ffsec× dc × c / g |
| dc |
n |
∇ |
tProp |
tAcc |
VCru |
dAcc |
dec.
comp. |
Mass
Mult. |
Daily
Diff. |
Prop.
time |
Accel
time |
Cruise
Veloc. |
Accel
Dist. |
| .300 | 1.05 | 0.90% GW | 38.2 days | 9.6 days | 4.6 AU/dy | 28 AU |
| .400 | 1.09 | 0.65% GW | 53.2 days | 13.3 days | 6.4 AU/dy | 43 AU |
| .500 | 1.15 | 0.49% GW | 70.5 days | 17.6 days | 8.4 AU/dy | 75 AU |
| .600 | 1.25 | 0.38% GW | 91.6 days | 22.9 days | 10.9 AU/dy | 126 AU |
| .700 | 1.40 | 0.29% GW | 119.9 days | 30.0 days | 14.1 AU/dy | 214 AU |
| .800 | 1.67 | 0.21% GW | 163.2 days | 40.8 days | 18.9 AU/dy | 392 AU |
| .866 | 2.00 | 0.16% GW | 212.7 days | 53.2 days | 24.2 AU/dy | 659 AU |
| Given | 1
√(1 - dc2) | .2826%GW
n× dc | log(1-%TOGW)
log(1-ε∇) | tProp
4 | c[1-RctAcc] |
|
|---|
Propulsion Time (tProp): Propulsion system converts fuel to energy; thus, propulsion time is the ship's range, available travel time (days) due to completely converting ship's available fuel to forward momentum.
--%TOGW: Relative amount of vessel's initial fuel is often called: "Percent Take Off Gross Weight (%TOGW)".
EXAMPLE-1: If vessel's initial GW is 100 mTs and initial fuel load is 60 mTs; then %TOGW is 60%, and ship's minimum weight is 40%TOGW or 40mT.
EXAMPLE-2: If %TOGW is 50%; then, ship's minimum weight (fuel depleted) is 50%. For convenience, this table assumes %TOGW = 50%.
--Daily Difference (∇): To maintain g-force propulsion, ship's exhaust requires a certain daily amount (∇) to exit ship's exhaust at certain high velocity to properly contribute to ship's forward momentum.
--Efficiency Coefficient (ε): To overcome inevitable inefficiencies, ship's daily fuel consumption must exceed ∇ to ensure g-force momentum. ε is TE's best guess as to how much to increase. For this table, TE conveniently assumes a single value of 2 for ε. For following tables, TE artificially assumes a dynamic efficiency model as described below.
Acceleration Time: Typical interstellar flight profile can divide into four phases; thus, P =4. Phases are described below; for each phase,: t = tProp/4.
Phase-1) Accelerate to midpoint. Fuel = GW0(1-(1-∇)t) = 15.9% TOGW
Phase-2) Decelerate to dest. Fuel = GW0(1-(1-∇)2t) = 29.3% TOGW
Phase-3) Accelerate back to midpoint. Fuel = GW0(1-(1-∇)3t) = 40.5% TOGW
Phase-4) Decelerate and return to dept. Fuel = GW0(1-(1-∇)4t) = 50.0% TOGW
AXIOMATIC: All four phases require equal time/distance.
Cruise Velocity: After t days of g-force propulsion, vessel accelerates to velocity which it will maintain throughout the cruise portion of flight to nearby star.
--Daily Light Speed Increment due to g-force (Δ): AXIOMATIC: After one day of g-force, the vessel accelerates to a velocity of 86,400 sec × 9.8065 m/s² = 847.2 km/sec = 0.2826% c = Δ . Due to relativity, TE further assumes that Δ = 0.2826% c for any g-force day.
--Daily Remainder till light speed (Rc): Thus, on-board observer measures c(1-Δ) = 0.997174 c, remaining velocity until light speed, c for any g-force day. TE assumes that earth-bound observer can model ship's velocity as difference between c ( 299,792,458 m/s = 173.145 AU/day) and recurring remainder (1-Δ)t which can be determined with exponential, t, as number of g-force days.
Acceleration Distance: TE uses a calculus textbook to determine a straightforward method to calculate distance from above velocity equation.
|
| E | 
Efficiency (E)
output divided by input.
It's always less than one.
Assume propulsion system super-heats some water into 1,000 plasma ions for the propulsion system. The goal is to output all 1,000 particles from the exhaust manifold at very high speeds and contribute to the ship's forward momentum. However, inevitable inefficiencies will prevent some of the 1,000 from fulfilling their destiny. EXAMPLE: If only 750 ions make it out the exhaust, efficiency is 75%:
E = out/in = 750/1000 = 75%.
|
|---|
| E' | Inefficiency (E') is loss divided by input.
In above example of the 1,000 water ions, 25% don't make to the exhaust.
Since total input equals output + loss; E + E' = 100%.
Thus, E and E' are complementary,
and we can determine a system's efficiency by measuring its inefficiency.
E = 1- E'
|
|---|
|
| E' |
TE assumes a model of decreasing inefficiency to enable a corresponding model of increasing efficiency. Assume that ever improving technology will increase propulsion performance while simultaneously decreasing inefficiency. TE arbitrarily assumes a baseline of 50% inefficiency when propulsion performance enables a mass multiple (n) of 2. For every integer increase above n = 2, TE arbitrarily assumes an inefficiency decrease of 10%: E' = (.5×.9n-2).Thus,
E = 1 - E' = 1 - (.5×.9n-2)
|
|---|
|
|---|
| ε |
Efficiency Factor is Inverse of Efficiency: ε = 1/E.
If you need 1,000 high speed ions to move vessel at g-force for one second, but systemic inefficiency only allows 750 ions through the propulsion system; then, the system needs to offset the input with enough ions to bring the output to the full 1,000. Thus, planners can use efficiency factor to to do this. Until experience gives us actual efficiency factor; arbitrarily assume:
|
|---|
|
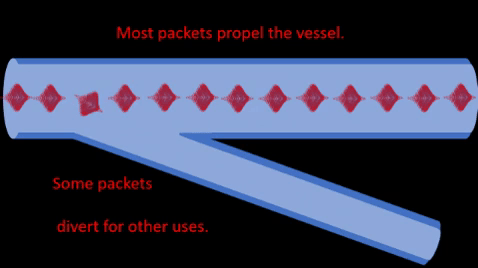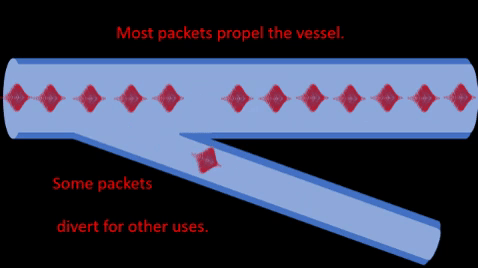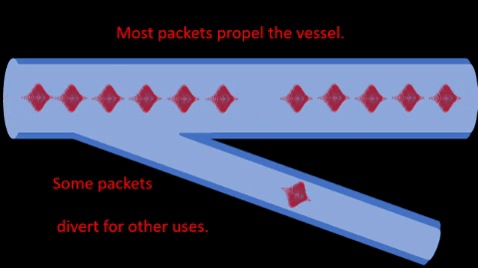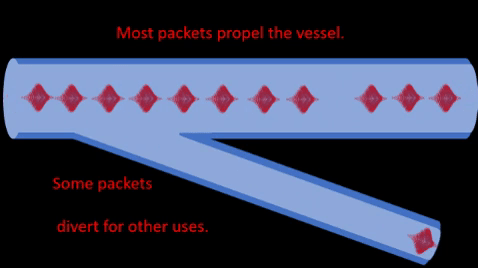
Let one second of consumed fuel flow, ffsec,
be 1,000 gms (one kilogram);
let it achieve a relativistic velocity, dc×c = .992c = 99.2% light speed;
then, 1.0 kg of ffsec will become 8.0 kgs of exhaust fuel flow, ffExh,
just before it exits vessel and contributes to forward momentum.
For convenience, note following identities:
| Dynamic Efficiency Factor (ε): As time passes, propulsion systems will likely improve with both better performance and better efficiency. If exhaust particle speed is a good performance measure; then, relativistic mass growth, n, is also a good measure since it maps directly to speed. Furthermore, Thought Experiment (TE) artificially assumes a dynamic efficiency model using same n value to reflect ever improving efficiency.
| Increases G-force Efficiency |
| ∇= | ffDay
MShip | = | 86,400sec×ffSec
n×ffSec×dc×c/g | = | .86,400×g/c
n × dc | = | .2826% GW
√(n2-1) |
|---|
|
| n |
∇ |
ε |
tAcc |
VCru |
dAcc |
Mass
Mult. |
Daily
Diff. |
Effic.
Fact. |
Accel
time |
Cruise
Vel. |
Accel
dist. |
| 2 | 0.163% | 2.00 | 53 days | 24.1 AU/dy | 655 AU |
| 3 | 0.100% | 1.82 | 95 days | 40.9 AU/dy | 2,038 AU |
| 4 | 0.073% | 1.68 | 141 days | 57.0 AU/dy | 4,295 AU |
| 5 | 0.058% | 1.57 | 191 days | 72.3 AU/dy | 7,510 AU |
| 6 | 0.048% | 1.49 | 244 days | 86.3 AU/dy | 11,709 AU |
| 7 | 0.041% | 1.42 | 299 days | 98.9 AU/dy | 16,870 AU |
| 8 | 0.036% | 1.36 | 357 days | 110.1 AU/dy | 22,939 AU |
| Given | .2826%
√(n2-1) | 1
1-(.5×.9n-2) | log(1-%TOGW)
4×log(1-ε∇) |
c[1-RctAcc]
|
|
Percent Take Off Gross Weight (%TOGW)explained in previous table.
For above table, TE assumes %TOGW= 50%.
Daily Remaining velocity (Rc) explained in previous table.
TE assumes on-board observer measures Rc = c(1-Δ) = 0.997174 c,
|
 |
Acceleration: After one year (365.25 days) of g-force propulsion, vessel will achieve velocity of 64.43% light speed (c). Vessel will maintain this velocity throughout entire cruise phase until it begins the deceleration phase.
Time: Acceleration duration is not entirely arbitrary. Vessel's range must accommodate acceleration to cruise, deceleration from cruise to destination and repeat for return leg of journey. Thus, one quarter of vessel's range can be considered available for acceleration duration.
Velocity: TE assumes cruise velocity determined by following equation:
VCru=c[1 - Rct]
Distance: TE assumes acceleration distance determined by following equation:
d = c[t + VCru/ln(Rc)]
Deceleration: To maintain g-force while decelerating from 64.43%c to essentially zero, deceleration requires same duration/distance as acceleration. |
|
Consistent Acceleration Time
| Destination | Acceleration
Time | Accel.
Velocity. |
Accel.
distance | Cruise
distance | Cruise
time | Total
Time |
|---|
| Star | D | t | tDay | VCru | d | dCru | tCru | tr | T |
|---|
| Name | LY | Yr | day | % c | LY | LY | Yrs | Yrs | Yrs |
|---|
| α Cent | 4.365 | 1.0 | 365.3 | 64.43% | 0.38 | 3.61 | 5.60 | 3.34 | 7.60 |
| Bar...Sta | 5.970 | 1.0 | 365.3 | 64.43% | 0.38 | 5.21 | 8.08 | 4.83 | 10.08 |
| Luh...16 | 6.600 | 1.0 | 365.3 | 64.43% | 0.38 | 5.85 | 9.07 | 5.41 | 11.07 |
| OBSERVED | Given | 365.25t | See previous tables. | D - 2d | dCru
VCru | tCru
n | tCru+2t |
|---|
Cruise: Due to vessel's finite range, interstellar vessel must stop acceleration well prior to the interstellar midpoint and maintain constant velocity until deceleration.
Cruise Velocity achieved during acceleration remains vessel's velocity during cruise duration.
Cruise Distance: determined by subtracting acceleration distance (d) and deceleration distance (d) from total trip distance (D). Since acceleration distance equals deceleration distance; cruise distance can be expressed:
dCru = D - 2 d
Cruise Time can be expressed as normal time and dilated time.
Normal
Time | At vessel velocity of 64.43%c,
Earth bound observer
measures 1.55 years
for each LY of cruise distance. |
|
|---|
Dilated
Time | Due to relativistic effects,
ship bound chronometer
measures:1.19 years
for each cruise LY. |
|
|---|
|
Tailor Acceleration Times
| Destination | Acceleration
Time | Accel.
Distance | Cruise
Velocity |
Cruise
Distance | Cruise
Time | Total
Time |
|---|
Star
Name | D | t | tDay | d | VCru | dCru | tCru | tr | T |
|---|
| LY | Yr | day | LY | % c | LY | Yrs | Yrs | Yrs |
| α Cent | 4.365 | 0.8 | 292.2 | 0.26 | 52.26% | 3.85 | 6.85 | 5.66 | 8.45 |
| Bar... Sta | 5.970 | 0.9 | 328.7 | 0.31 | 60.56% | 5.34 | 8.82 | 7.02 | 10.62 |
| Luh...16 | 6.600 | 1.0 | 365.3 | 0.38 | 64.43% | 5.85 | 9.07 | 6.94 | 11.07 |
| Wolf..359 | 7.786 | 1.1 | 401.8 | 0.40 | 67.92% | 6.90 | 10.16 | 7.46 | 12.36 |
| Lal...21185 | 8.291 | 1.2 | 438.3 | 0.51 | 71.07% | 7.27 | 10.22 | 7.19 | 12.62 |
| Sirius | 8.58 | 1.3 | 474.8 | 0.58 | 73.91% | 7.41 | 10.03 | 6.76 | 12.63 |
| OBSERVED | Given | 365.25t | See previous tables | D - 2d | dCru
VCru | tCru
n | tCru+2t |
|---|
NOTE-1: Arbitrarily select some nearby stars and arrange from nearest to farthest.
NOTE-2: Choose acceleration times; start low and increment as shown.
As destination distances increase, consider increasing acceleration times to increase cruise velocities. Thus, overall trip time would decrease. |
 Some 52 neighboring stellar systems now lie within about 15 light-years of Sol, our Sun. These contain a total of 63 stars; several systems contain multiple stars (i.e., binary or tertiary systems). Also, we now know of 11 brown dwarfs (not quite massive enough to fuse hydrogen) and four white dwarfs (extremely dense collapsed cores). Despite their relative proximity, only nine of above objects are visible to a typical naked eye on Earth. |
| LONGER PROPULSION TIMES means accelerating to a faster cruise velocity which reduces cruise time which leads to shorter overall trip time. | SHORTER PROPULSION TIMES
accelerate the vessel to a slower cruise velocity;
thus, cruise duration increases.
However, it requires less fuel
which decreases risk of in-flight fuel depletion.
WARNING: If ship runs out of fuel at a high velocity; then, vessel and occupants are stranded at that velocity with no way to slow down. |
Tailor Propulsion Performance
to accommodate destination distances. Increase mass multiple (n)
to increase acceleration time and decrease cruise time.
| n | dc | ∇ | ε | Accel.
Time (t) | Cruise
Vel. (VCru) | Accel.
Dist. (d) | | 8 | 0.9922 | 0.036%GW | 1.36 | 357 dy | 0.98Yr | 110.2AU/dy | .636c | 22,939AU | 0.36LY |
| 9 | 0.9938 | 0.032%GW | 1.31 | 417 dy | 1.14Yr | 120,0AU/dy | .693c | 29,842AU | 0.47LY | | 10 | 0.9950 | 0.028%GW | 1.27 | 479 dy | 1.31Yr | 128.5AU/dy | .742c | 37,490AU | 0.59LY | | 11 | 0.9959 | 0.026%GW | 1.24 | 542 dy | 1.48Yr | 135.8AU/dy | .784c | 45,793AU | 0.72LY | | 12 | 0.9965 | 0.024%GW | 1.21 | 605 dy | 1.66Yr | 141.9AU/dy | .820c | 54,661AU | 0.86LY | | 13 | 0.9970 | 0.022%GW | 1.19 | 670 dy | 1.83Yr | 147.2AU/dy | .850c | 64,010AU | 1.01LY | | 14 | 0.9974 | 0.020%GW | 1.16 | 735 dy | 2.01Yr | 151.5AU/dy | .875c | 73,763AU | 1.17LY | | 15 | 0.9978 | 0.019%GW | 1.15 | 801 dy | 2.19Yr | 155.2AU/dy | .896c | 83,850AU | 1.33LY | | Given | √(n2-1)
n | 0.2826%
√(n2-1) | 1
1-(.5×.9n-2) | log(1-%TOGW)
4×log(1-ε∇) | c[1 - Rct] | |
| | Destination | Mass
Mult | Accel
time | Cruise
Vel. | Accel.
dist. | Cruise
dist. | Cruise
Time | Total
Time | | Star | D | n | t | VCru | d | dCru | tCru | tr | T | | Name | LY | Yr | dec. c | LY | LY | Yr | Yr | Yr |
|---|
| α Cent | 4.365 | 8 | 0.98 | 0.64 c | 0.36 | 2.42 | 3.80 | 2.93 | 5.65 | | Bar...Sta | 5.970 | 9 | 1.14 | 0.69 c | 0.47 | 3.69 | 5.33 | 3.84 | 7.61 | | Luh...16 | 6.600 | 10 | 1.31 | 0.74 c | 0.59 | 3.99 | 5.37 | 3.60 | 7.99 | | Wolf..359 | 7.786 | 11 | 1.48 | 0.78 c | 0.72 | 4.83 | 6.16 | 3.82 | 9.13 | | Lal...21185 | 8.291 | 12 | 1.66 | 0.82 c | 0.86 | 4.99 | 6.08 | 3.48 | 9.40 | | Sirius | 8.583 | 13 | 1.83 | 0.85 c | 1.01 | 4.92 | 5.79 | 3.05 | 9.46 | | Lu.726-8 | 8.728 | 14 | 2.01 | 0.88 c | 1.17 | 4.71 | 5.38 | 2.60 | 9.41 | | Ross 154 | 9.681 | 15 | 2.19 | 0.90 c | 1.33 | 5.30 | 5.92 | 2.62 | 10.30 | | OBSERVED | See green table in left panel. | D - 2d | dCru
VCru | tCru
nCru | tCru +2t |
|---|
|
| CONCLUSION: Many ways to get there!!! |
|---|
|
|
0 Comments:
Post a Comment
<< Home